Page 226 - Start Up Mathematics_8 (Non CCE)

Solution: (a) GUNS is a parallelogram, therefore,
SN = GU and SG = NU (Opposite sides of a parallelogram are equal)

fi 3y – 1 = 26 and 3x = 18
18
fi 3y = 27 and x = = 6
3
fi y = 9 cm and x = 6 cm
(b) RUNS is a parallelogram, therefore,
ON = OR and OS = OU (Diagonals of a parallelogram bisect each other)

fi x + y = 16 and y + 7 = 20
fi x + y = 16 and y = 20 – 7 = 13
fi x + 13 = 16 fi x = 16 – 13 = 3
fi x = 3 cm and y = 13 cm
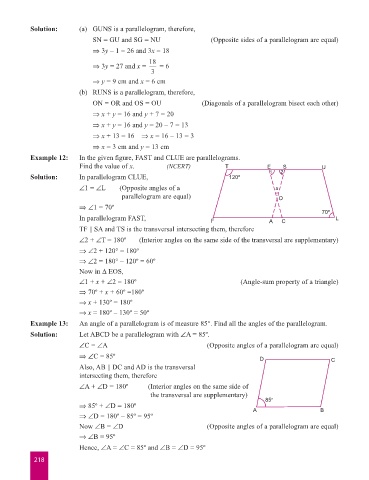
Example 12: In the given figure, FAST and CLUE are parallelograms.
Find the value of x. (NCERT) T E S U
1 2
Solution: In parallelogram CLUE, 120º
–1 = –L ( Opposite angles of a x
parallelogram are equal) O

fi –1 = 70º
70º
In parallelogram FAST, F A C L
TF || SA and TS is the transversal intersecting them, therefore
–2 + –T = 180º (Interior angles on the same side of the transversal are supplementary)

fi –2 + 120° = 180º
fi –2 = 180° – 120º = 60º
Now in D EOS,
–1 + x + –2 = 180º (Angle-sum property of a triangle)
fi 70º + x + 60º =180º
fi x + 130º = 180º
fi x = 180º – 130º = 50º
Example 13: An angle of a parallelogram is of measure 85°. Find all the angles of the parallelogram.

Solution: Let ABCD be a parallelogram with –A = 85º.
–C = –A (Opposite angles of a parallelogram are equal)
fi –C = 85º D C
Also, AB || DC and AD is the transversal
intersecting them, therefore
–A + –D = 180º ( Interior angles on the same side of
the transversal are supplementary)
85º
fi 85º + –D = 180º A B
fi –D = 180º – 85º = 95º
Now –B = –D (Opposite angles of a parallelogram are equal)

fi –B = 95º
Hence, –A = –C = 85º and –B = –D = 95º
218