Page 225 - Start Up Mathematics_8 (Non CCE)

EF || DG and EG is the transversal intersecting them, therefore,
–FEG = –DGE (Alternate interior angles)

fi y = z fi z = 60º
Therefore, x = 90º, y = z = 60º
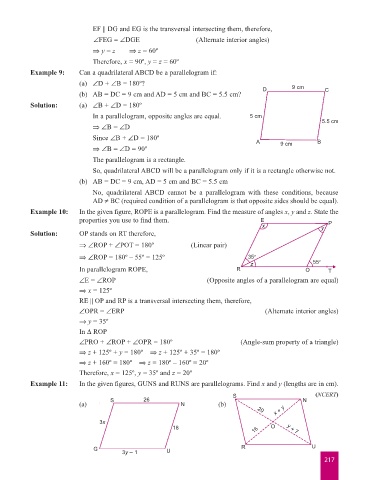
Example 9: Can a quadrilateral ABCD be a parallelogram if:
(a) –D + –B = 180º?
D 9 cm C
(b) AB = DC = 9 cm and AD = 5 cm and BC = 5.5 cm?
Solution: (a) –B + –D = 180º
In a parallelogram, opposite angles are equal. 5 cm
5.5 cm
fi –B = –D
Since –B + –D = 180º
A 9 cm B
fi –B = –D = 90º
The parallelogram is a rectangle.
So, quadrilateral ABCD will be a parallelogram only if it is a rectangle otherwise not.
(b) AB = DC = 9 cm, AD = 5 cm and BC = 5.5 cm

No, quadrilateral ABCD cannot be a parallelogram with these conditions, because
AD π BC (required condition of a parallelogram is that opposite sides should be equal).

Example 10: In the given figure, ROPE is a parallelogram. Find the measure of angles x, y and z. State the
properties you use to find them. E P
x y
Solution: OP stands on RT therefore,
fi –ROP + –POT = 180º (Linear pair)

fi –ROP = 180º – 55º = 125º 35º
z 55º
In parallelogram ROPE, R O T

–E = –ROP (Opposite angles of a parallelogram are equal)
fi x = 125º
RE || OP and RP is a transversal intersecting them, therefore,
–OPR = –ERP (Alternate interior angles)
fi y = 35º
In D ROP
–PRO + –ROP + –OPR = 180º (Angle-sum property of a triangle)
fi z + 125º + y = 180º fi z + 125º + 35º = 180º
fi z + 160º = 180º fi z = 180º – 160º = 20º
Therefore, x = 125º, y = 35º and z = 20º

Example 11: In the given figures, GUNS and RUNS are parallelograms. Find x and y (lengths are in cm).
S (NCERT)
(a) S 26 N (b) N
x + y
20
3x
18 16 O y + 7

G U R U
3y – 1
217