Page 223 - Start Up Mathematics_8 (Non CCE)

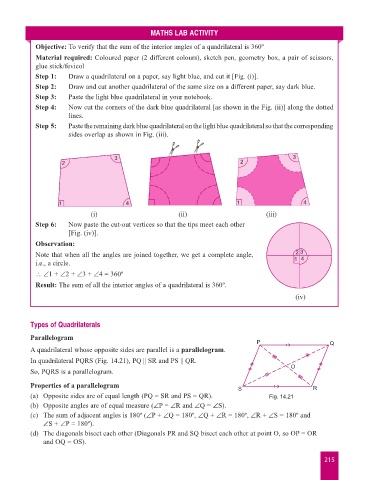
MATHS LAB ACTIVITY

Objective: To verify that the sum of the interior angles of a quadrilateral is 360º
Material required: Coloured paper (2 different colours), sketch pen, geometry box, a pair of scissors,
glue stick/fevicol
Step 1: Draw a quadrilateral on a paper, say light blue, and cut it [Fig. (i)].
Step 2: Draw and cut another quadrilateral of the same size on a different paper, say dark blue.
Step 3: Paste the light blue quadrilateral in your notebook.
Step 4: Now cut the corners of the dark blue quadrilateral [as shown in the Fig. (ii)] along the dotted
lines.
Step 5: Paste the remaining dark blue quadrilateral on the light blue quadrilateral so that the corresponding
sides overlap as shown in Fig. (iii).

3 3
2 2

1 4 1 4
(i) (ii) (iii)
Step 6: Now paste the cut-out vertices so that the tips meet each other
[Fig. (iv)].
Observation:
3
2
Note that when all the angles are joined together, we get a complete angle, 1 4
i.e., a circle.
∴ ∠1 + ∠2 + ∠3 + ∠4 = 360º
Result: The sum of all the interior angles of a quadrilateral is 360º.

(iv)

Types of Quadrilaterals
Parallelogram
P Q
A quadrilateral whose opposite sides are parallel is a parallelogram.
In quadrilateral PQRS (Fig. 14.21), PQ || SR and PS || QR.
O
So, PQRS is a parallelogram.
Properties of a parallelogram S R
(a) Opposite sides are of equal length (PQ = SR and PS = QR). Fig. 14.21
(b) Opposite angles are of equal measure (–P = –R and –Q = –S).
(c) The sum of adjacent angles is 180º (–P + –Q = 180º, –Q + –R = 180º, –R + –S = 180º and
–S + –P = 180º).
(d) The diagonals bisect each other (Diagonals PR and SQ bisect each other at point O, so OP = OR
and OQ = OS).

215