Page 228 - Start Up Mathematics_8 (Non CCE)

Properties of a square
(a) All sides are of equal length (PQ = QR = SR = SP).
(b) All interior angles measure 90º (–P = –Q = –R = –S = 90º).
(c) Diagonals are of equal length (PR = SQ).
(d) Diagonals bisect each other at right angles (OP = OR and OQ = OS, also PR ^ SQ at point O). Hence,
OP = OR = OQ = OS.

Example 14: Explain how a square is a: (a) quadrilateral, (b) parallelogram, (c) rhombus, (d) rectangle.
(NCERT)
Solution: (a) Square is a quadrilateral because it is a closed figure with four sides and four angles.
(b) Square is a parallelogram because its opposite sides are parallel.
(c) Square is a rhombus because all its sides are equal and the diagonals bisect each other
at right angles.
(d) Square is a rectangle because all its angles are of 90º and the diagonals are equal in
length. D E
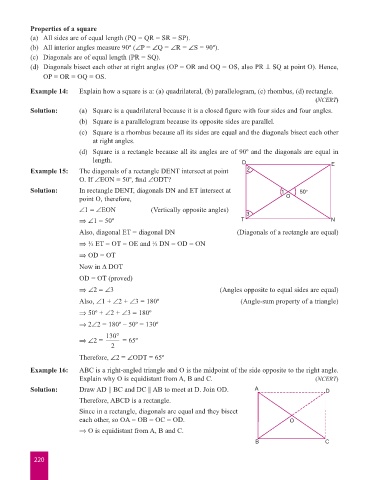
Example 15: The diagonals of a rectangle DENT intersect at point 2
O. If –EON = 50º, find –ODT?
Solution: In rectangle DENT, diagonals DN and ET intersect at 1 50º
point O, therefore, O
–1 = –EON (Vertically opposite angles)
3
fi –1 = 50º T N

Also, diagonal ET = diagonal DN (Diagonals of a rectangle are equal)
fi ½ ET = OT = OE and ½ DN = OD = ON
fi OD = OT

Now in D DOT
OD = OT (proved)

fi –2 = –3 (Angles opposite to equal sides are equal)
Also, –1 + –2 + –3 = 180º (Angle-sum property of a triangle)
fi 50º + –2 + –3 = 180º
fi 2–2 = 180º – 50º = 130º

130∞
fi –2 = = 65º
2
Therefore, –2 = –ODT = 65º

Example 16: ABC is a right-angled triangle and O is the midpoint of the side opposite to the right angle.
Explain why O is equidistant from A, B and C. (NCERT)
Solution: Draw AD || BC and DC || AB to meet at D. Join OD. A D
Therefore, ABCD is a rectangle.
Since in a rectangle, diagonals are equal and they bisect
each other, so OA = OB = OC = OD. O
fi O is equidistant from A, B and C.

B C

220