Page 229 - Start Up Mathematics_8 (Non CCE)

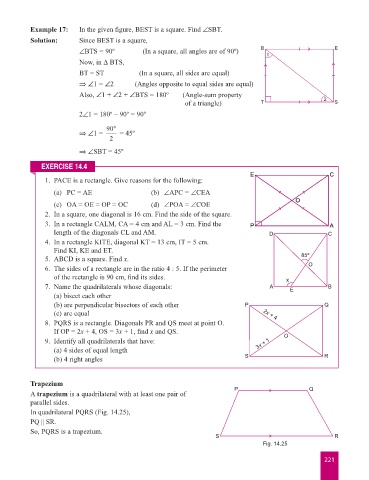
Example 17: In the given figure, BEST is a square. Find –SBT.

Solution: Since BEST is a square,
–BTS = 90º (In a square, all angles are of 90º) B E
1
Now, in D BTS,
BT = ST (In a square, all sides are equal)

fi –1 = –2 (Angles opposite to equal sides are equal)
Also, –1 + –2 + –BTS = 180º ( Angle-sum property
of a triangle) T 2 S
2–1 = 180º – 90º = 90º

90∞
fi –1 = = 45º
2
fi –SBT = 45º

EXERCISE 14.4

1. PACE is a rectangle. Give reasons for the following:

(a) PC = AE (b) ∠APC = ∠CEA
(c) OA = OE = OP = OC (d) ∠POA = ∠COE
2. In a square, one diagonal is 16 cm. Find the side of the square.
3. In a rectangle CALM, CA = 4 cm and AL = 3 cm. Find the
length of the diagonals CL and AM. D C
4. In a rectangle KITE, diagonal KT = 13 cm, IT = 5 cm.
Find KI, KE and ET.
5. ABCD is a square. Find x. 85º
6. The sides of a rectangle are in the ratio 4 : 5. If the perimeter O
of the rectangle is 90 cm, find its sides. x
7. Name the quadrilaterals whose diagonals: A E B
(a) bisect each other
(b) are perpendicular bisectors of each other P Q
(c) are equal 2x + 4
8. PQRS is a rectangle. Diagonals PR and QS meet at point O.
If OP = 2x + 4, OS = 3x + 1, find x and QS.
9. Identify all quadrilaterals that have: + 1 O
(a) 4 sides of equal length 3x
(b) 4 right angles S R

Trapezium
A trapezium is a quadrilateral with at least one pair of P Q
parallel sides.
In quadrilateral PQRS (Fig. 14.25),
PQ || SR.
So, PQRS is a trapezium.
S R
Fig. 14.25

221