Page 227 - Start Up Mathematics_8 (Non CCE)

EXERCISE 14.3

1. Two adjacent angles of parallelogram PQRS are in the ratio 2 : 7. Find all the angles of the parallelogram.
2. If an angle of a parallelogram is two-third of its adjacent angle, find the angles of the parallelogram.
3. The shorter side of a parallelogram is 3.6 cm and the longer side is half as much again as the shorter
side. Find the perimeter of the parallelogram.

4. One angle of the parallelogram is 70º. Find all the other angles of the parallelogram.
5. In the parallelogram SPUN, ∠P = 3∠S. Find all the angles of the parallelogram.
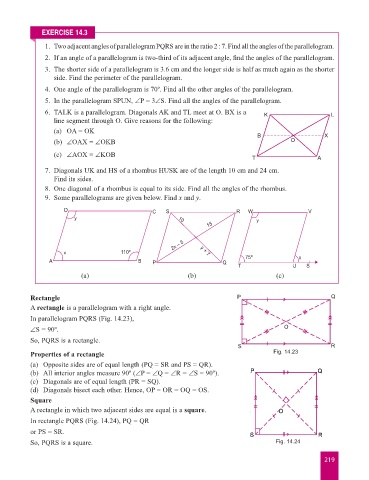
6. TALK is a parallelogram. Diagonals AK and TL meet at O. BX is a K L
line segment through O. Give reasons for the following:
(a) OA = OK
B X
(b) ∠OAX = ∠OKB O

(c) ∠AOX = ∠KOB
T A
7. Diagonals UK and HS of a rhombus HUSK are of the length 10 cm and 24 cm.
Find its sides.
8. One diagonal of a rhombus is equal to its side. Find all the angles of the rhombus.
9. Some parallelograms are given below. Find x and y.

D C S R W V
y 10 y
15

– 5
2x
x 110º y + 7
A B P Q 75º x
T U S
(a) (b) (c)

Rectangle P Q
A rectangle is a parallelogram with a right angle.
In parallelogram PQRS (Fig. 14.23),

–S = 90º. O
So, PQRS is a rectangle.
S R
Properties of a rectangle Fig. 14.23
(a) Opposite sides are of equal length (PQ = SR and PS = QR).
(b) All interior angles measure 90º (–P = –Q = –R = –S = 90º).
(c) Diagonals are of equal length (PR = SQ).
(d) Diagonals bisect each other. Hence, OP = OR = OQ = OS.
Square
A rectangle in which two adjacent sides are equal is a square.
In rectangle PQRS (Fig. 14.24), PQ = QR
or PS = SR.

So, PQRS is a square. Fig. 14.24

219