Page 218 - Start Up Mathematics_8 (Non CCE)

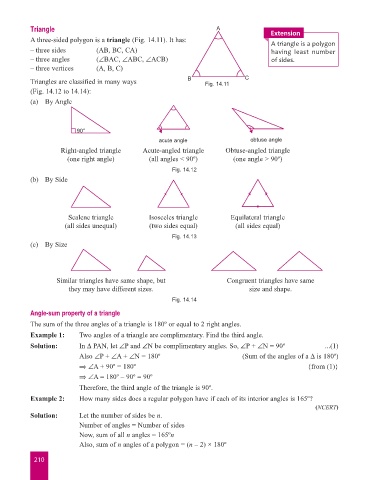
Triangle A Extension
A three-sided polygon is a triangle (Fig. 14.11). It has: A triangle is a polygon

– three sides (AB, BC, CA) having least number
– three angles (–BAC, –ABC, –ACB) of sides.
– three vertices (A, B, C)

Triangles are classified in many ways B Fig. 14.11 C
(Fig. 14.12 to 14.14):
(a) By Angle

90°
acute angle obtuse angle
Right-angled triangle Acute-angled triangle Obtuse-angled triangle
(one right angle) (all angles < 90º) (one angle > 90º)
Fig. 14.12
(b) By Side

Scalene triangle Isosceles triangle Equilateral triangle
(all sides unequal) (two sides equal) (all sides equal)

Fig. 14.13
(c) By Size

Similar triangles have same shape, but Congruent triangles have same
they may have different sizes. size and shape.

Fig. 14.14
Angle-sum property of a triangle
The sum of the three angles of a triangle is 180º or equal to 2 right angles.

Example 1: Two angles of a triangle are complimentary. Find the third angle.
Solution: In D PAN, let –P and –N be complimentary angles. So, –P + –N = 90º ...(1)
Also –P + –A + –N = 180º (Sum of the angles of a D is 180º)
fi –A + 90º = 180º {from (1)}
fi –A = 180º – 90º = 90º

Therefore, the third angle of the triangle is 90º.
Example 2: How many sides does a regular polygon have if each of its interior angles is 165º?
(NCERT)
Solution: Let the number of sides be n.
Number of angles = Number of sides
Now, sum of all n angles = 165ºn
Also, sum of n angles of a polygon = (n – 2) × 180º

210