Page 151 - Start Up Mathematics_7

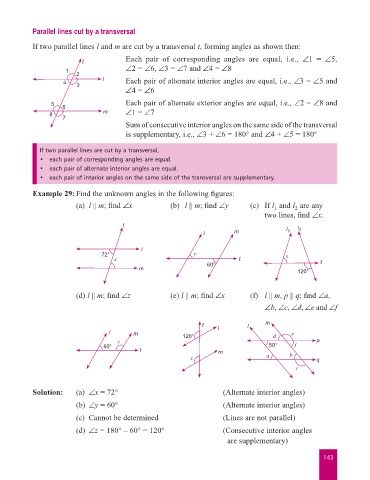
Parallel lines cut by a transversal
If two parallel lines l and m are cut by a transversal t, forming angles as shown then:

Each pair of corresponding angles are equal, i.e., ∠1 = ∠5,
t
1 ∠2 = ∠6, ∠3 = ∠7 and ∠4 = ∠8
2
4 3 l Each pair of alternate interior angles are equal, i.e., ∠3 = ∠5 and
∠4 = ∠6

5 6 Each pair of alternate exterior angles are equal, i.e., ∠2 = ∠8 and
8 m ∠1 = ∠7
7
Sum of consecutive interior angles on the same side of the transversal
is supplementary, i.e., ∠3 + ∠6 = 180° and ∠4 + ∠5 = 180°

If two parallel lines are cut by a transversal,
• each pair of corresponding angles are equal.
• each pair of alternate interior angles are equal.
• each pair of interior angles on the same side of the transversal are supplementary.

Example 29: Find the unknown angles in the following figures:
(a) l || m; find ∠x (b) l || m; find ∠y (c) If l and l are any
2
1
two lines, find ∠x.
t l l
l m 1 2

l
72° y x
x t
60° t
m
120°

(d) l || m; find ∠z (e) l || m; find ∠x (f) l || m, p || q; find ∠a,
∠b, ∠c, ∠d, ∠e and ∠f

t l l m
l m 120° d e
z p
60° 50° f
t m
x a b q
c

Solution: (a) ∠x = 72° (Alternate interior angles)
(b) ∠y = 60° (Alternate interior angles)

(c) Cannot be determined (Lines are not parallel)

(d) ∠z = 180° – 60° = 120° (Consecutive interior angles
are supplementary)

143