Page 154 - Start Up Mathematics_7

Solution: (a) The complement of ∠ABD is ∠DBC.
(b) The complement of ∠BDE is ∠DBE.

(c) The supplement of ∠ADE is ∠EDC.

(d) AB || DE,  ∠ABE + ∠DEB = 180°, i.e., sum of cointerior angles is supplementary.

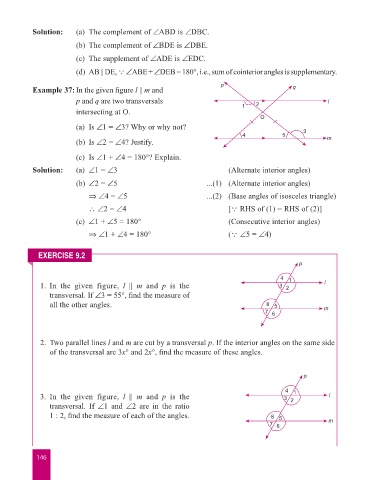
p
Example 37: In the given figure l || m and q
p and q are two transversals 1 2 l
intersecting at O.
O
(a) Is ∠1 = ∠3? Why or why not?
4 5 3 m
(b) Is ∠2 = ∠4? Justify.

(c) Is ∠1 + ∠4 = 180°? Explain.

Solution: (a) ∠1 = ∠3 (Alternate interior angles)

(b) ∠2 = ∠5 ...(1) (Alternate interior angles)
⇒ ∠4 = ∠5 ...(2) (Base angles of isosceles triangle)

∴ ∠2 = ∠4 [ RHS of (1) = RHS of (2)]

(c) ∠1 + ∠5 = 180° (Consecutive interior angles)
⇒ ∠1 + ∠4 = 180° ( ∠5 = ∠4)

EXERCISE 9.2
p

4 1
1. In the given figure, l || m and p is the 3 2 l
transversal. If ∠3 = 55°, find the measure of
all the other angles. 8 5
7 6 m

2. Two parallel lines l and m are cut by a transversal p. If the interior angles on the same side
of the transversal are 3x° and 2x°, find the measure of these angles.

p

4 1
3. In the given figure, l || m and p is the 3 2 l
transversal. If ∠1 and ∠2 are in the ratio
1 : 2, find the measure of each of the angles. 8 5
7 6 m

146