Page 156 - Start Up Mathematics_7

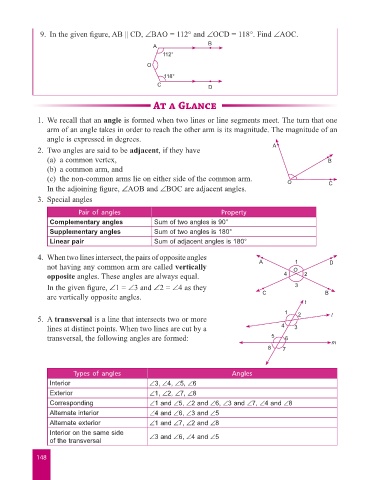
9. In the given figure, AB || CD, ∠BAO = 112° and ∠OCD = 118°. Find ∠AOC.

A B
112°

O
118°
C D

At a Glance

1. We recall that an angle is formed when two lines or line segments meet. The turn that one
arm of an angle takes in order to reach the other arm is its magnitude. The magnitude of an
angle is expressed in degrees.
2. Two angles are said to be adjacent, if they have A
(a) a common vertex, B
(b) a common arm, and
(c) the non-common arms lie on either side of the common arm. O
In the adjoining figure, ∠AOB and ∠BOC are adjacent angles. C
3. Special angles

Pair of angles Property
Complementary angles Sum of two angles is 90°
Supplementary angles Sum of two angles is 180°
Linear pair Sum of adjacent angles is 180°

4. When two lines intersect, the pairs of opposite angles
1
not having any common arm are called vertically A O D
opposite angles. These angles are always equal. 4 2
In the given figure, ∠1 = ∠3 and ∠2 = ∠4 as they 3
are vertically opposite angles. C B
t
1 2 l
5. A transversal is a line that intersects two or more
lines at distinct points. When two lines are cut by a 4 3
transversal, the following angles are formed: 5 6
m
8 7

Types of angles Angles
Interior ∠3, ∠4, ∠5, ∠6
Exterior ∠1, ∠2, ∠7, ∠8
Corresponding ∠1 and ∠5, ∠2 and ∠6, ∠3 and ∠7, ∠4 and ∠8
Alternate interior ∠4 and ∠6, ∠3 and ∠5
Alternate exterior ∠1 and ∠7, ∠2 and ∠8
Interior on the same side ∠3 and ∠6, ∠4 and ∠5
of the transversal

148