Page 150 - Start Up Mathematics_7

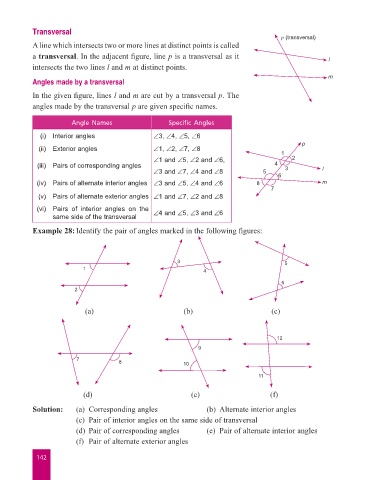
Transversal
p (transversal)
A line which intersects two or more lines at distinct points is called
a transversal. In the adjacent figure, line p is a transversal as it l

intersects the two lines l and m at distinct points.
m
Angles made by a transversal

In the given figure, lines l and m are cut by a transversal p. The
angles made by the transversal p are given specific names.

Angle Names Specific Angles

(i) Interior angles ∠3, ∠4, ∠5, ∠6
p
(ii) Exterior angles ∠1, ∠2, ∠7, ∠8
1
∠1 and ∠5, ∠2 and ∠6, 2
(iii) Pairs of corresponding angles 4 3 l
∠3 and ∠7, ∠4 and ∠8 5
6
(iv) Pairs of alternate interior angles ∠3 and ∠5, ∠4 and ∠6 8 m
7
(v) Pairs of alternate exterior angles ∠1 and ∠7, ∠2 and ∠8
(vi) Pairs of interior angles on the
same side of the transversal ∠4 and ∠5, ∠3 and ∠6
Example 28: Identify the pair of angles marked in the following figures:

3 5
1 4

6
2

(a) (b) (c)

12
9
7 8 10

11

(d) (e) (f)

Solution: (a) Corresponding angles (b) Alternate interior angles

(c) Pair of interior angles on the same side of transversal
(d) Pair of corresponding angles (e) Pair of alternate interior angles
(f) Pair of alternate exterior angles

142