Page 146 - Start Up Mathematics_7

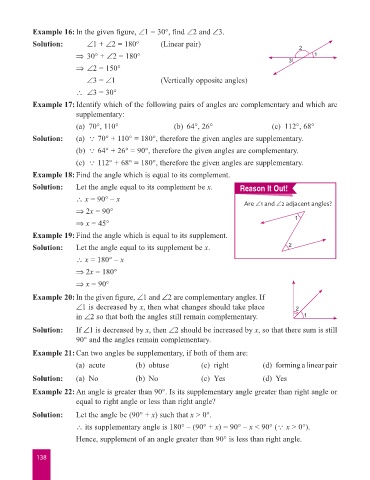
Example 16: In the given figure, ∠1 = 30°, find ∠2 and ∠3.
Solution: ∠1 + ∠2 = 180° (Linear pair)
2
⇒ 30° + ∠2 = 180° 1
3
⇒ ∠2 = 150°

∠3 = ∠1 (Vertically opposite angles)
∴ ∠3 = 30°
Example 17: Identify which of the following pairs of angles are complementary and which are
supplementary:

(a) 70°, 110° (b) 64°, 26° (c) 112°, 68°
Solution: (a)  70° + 110° = 180°, therefore the given angles are supplementary.

(b)  64° + 26° = 90°, therefore the given angles are complementary.
(c)  112° + 68° = 180°, therefore the given angles are supplementary.

Example 18: Find the angle which is equal to its complement.

Solution: Let the angle equal to its complement be x. Reason It Out!
∴ x = 90° – x
Are ∠1 and ∠2 adjacent angles?
⇒ 2x = 90°
1
⇒ x = 45°
Example 19: Find the angle which is equal to its supplement.

Solution: Let the angle equal to its supplement be x. 2
∴ x = 180° – x

⇒ 2x = 180°
⇒ x = 90°

Example 20: In the given figure, ∠1 and ∠2 are complementary angles. If
∠1 is decreased by x, then what changes should take place 2
in ∠2 so that both the angles still remain complementary. 1

Solution: If ∠1 is decreased by x, then ∠2 should be increased by x, so that there sum is still
90° and the angles remain complementary.

Example 21: Can two angles be supplementary, if both of them are:
(a) acute (b) obtuse (c) right (d) forming a linear pair

Solution: (a) No (b) No (c) Yes (d) Yes

Example 22: An angle is greater than 90°. Is its supplementary angle greater than right angle or
equal to right angle or less than right angle?
Solution: Let the angle be (90° + x) such that x > 0°.

∴ its supplementary angle is 180° – (90° + x) = 90° – x < 90° ( x > 0°).
Hence, supplement of an angle greater than 90° is less than right angle.

138