Page 144 - Start Up Mathematics_7

Example 8: Can two obtuse angles be supplement of each other?
Solution: Since the sum of two obtuse angles is greater than 180°, they can never be supplement
of each other.

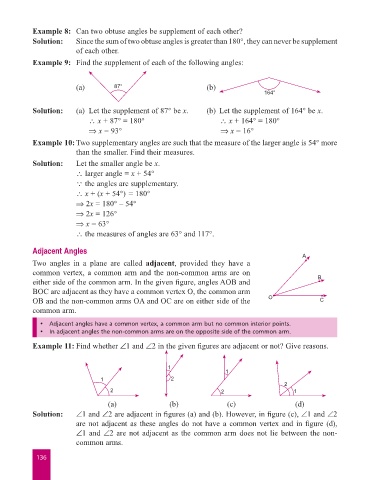
Example 9: Find the supplement of each of the following angles:

(a) 87° (b)
164°

Solution: (a) Let the supplement of 87° be x. (b) Let the supplement of 164° be x.
∴ x + 87° = 180° ∴ x + 164° = 180°
⇒ x = 93° ⇒ x = 16°
Example 10: Two supplementary angles are such that the measure of the larger angle is 54° more
than the smaller. Find their measures.

Solution: Let the smaller angle be x.
∴ larger angle = x + 54°
 the angles are supplementary.
∴ x + (x + 54°) = 180°
⇒ 2x = 180° – 54°
⇒ 2x = 126°
⇒ x = 63°
∴ the measures of angles are 63° and 117°.

Adjacent Angles
A
Two angles in a plane are called adjacent, provided they have a
common vertex, a common arm and the non-common arms are on
either side of the common arm. In the given figure, angles AOB and B
BOC are adjacent as they have a common vertex O, the common arm
OB and the non-common arms OA and OC are on either side of the O C
common arm.

• Adjacent angles have a common vertex, a common arm but no common interior points.
• In adjacent angles the non-common arms are on the opposite side of the common arm.
Example 11: Find whether ∠1 and ∠2 in the given figures are adjacent or not? Give reasons.

1
1
1 2
2
2 2 1

(a) (b) (c) (d)
Solution: ∠1 and ∠2 are adjacent in figures (a) and (b). However, in figure (c), ∠1 and ∠2
are not adjacent as these angles do not have a common vertex and in figure (d),
∠1 and ∠2 are not adjacent as the common arm does not lie between the non-
common arms.

136