Page 145 - Start Up Mathematics_7

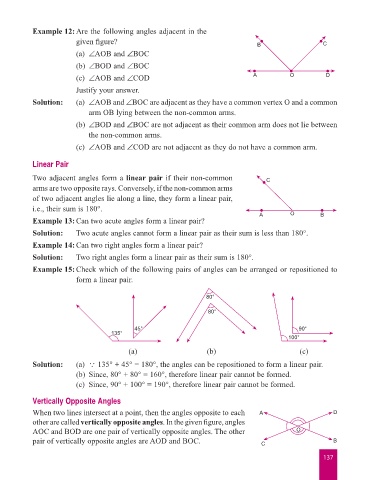
Example 12: Are the following angles adjacent in the

given figure? B C
(a) ∠AOB and ∠BOC
(b) ∠BOD and ∠BOC

(c) ∠AOB and ∠COD A O D

Justify your answer.
Solution: (a) ∠AOB and ∠BOC are adjacent as they have a common vertex O and a common
arm OB lying between the non-common arms.

(b) ∠BOD and ∠BOC are not adjacent as their common arm does not lie between
the non-common arms.
(c) ∠AOB and ∠COD are not adjacent as they do not have a common arm.

Linear Pair

Two adjacent angles form a linear pair if their non-common C
arms are two opposite rays. Conversely, if the non-common arms
of two adjacent angles lie along a line, they form a linear pair,
i.e., their sum is 180°.
A O B
Example 13: Can two acute angles form a linear pair?
Solution: Two acute angles cannot form a linear pair as their sum is less than 180°.

Example 14: Can two right angles form a linear pair?
Solution: Two right angles form a linear pair as their sum is 180°.
Example 15: Check which of the following pairs of angles can be arranged or repositioned to

form a linear pair.

80°

80°

45° 90°
135°
100°
(a) (b) (c)

Solution: (a)  135° + 45° = 180°, the angles can be repositioned to form a linear pair.
(b) Since, 80° + 80° = 160°, therefore linear pair cannot be formed.
(c) Since, 90° + 100° = 190°, therefore linear pair cannot be formed.

Vertically Opposite Angles
When two lines intersect at a point, then the angles opposite to each A D
other are called vertically opposite angles. In the given figure, angles
AOC and BOD are one pair of vertically opposite angles. The other O
pair of vertically opposite angles are AOD and BOC. C B

137