Page 148 - Start Up Mathematics_7

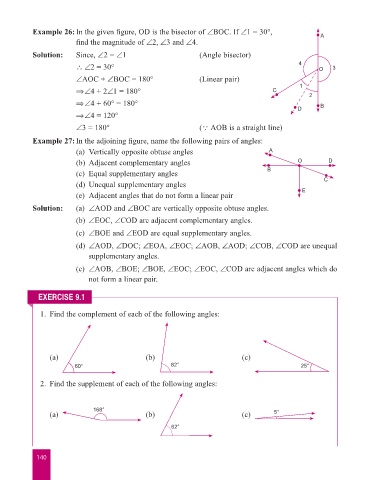
Example 26: In the given figure, OD is the bisector of ∠BOC. If ∠1 = 30°, A
find the magnitude of ∠2, ∠3 and ∠4.
Solution: Since, ∠2 = ∠1 (Angle bisector)

∴ ∠2 = 30° 4 O 3
∠AOC + ∠BOC = 180° (Linear pair)
1
⇒ ∠4 + 2∠1 = 180° C
2
⇒ ∠4 + 60° = 180°
D B
⇒ ∠4 = 120°
∠3 = 180° ( AOB is a straight line)

Example 27: In the adjoining figure, name the following pairs of angles:
(a) Vertically opposite obtuse angles A
(b) Adjacent complementary angles O D

(c) Equal supplementary angles B
(d) Unequal supplementary angles C
(e) Adjacent angles that do not form a linear pair E

Solution: (a) ∠AOD and ∠BOC are vertically opposite obtuse angles.
(b) ∠EOC, ∠COD are adjacent complementary angles.

(c) ∠BOE and ∠EOD are equal supplementary angles.

(d) ∠AOD, ∠DOC; ∠EOA, ∠EOC; ∠AOB, ∠AOD; ∠COB, ∠COD are unequal
supplementary angles.
(e) ∠AOB, ∠BOE; ∠BOE, ∠EOC; ∠EOC, ∠COD are adjacent angles which do
not form a linear pair.

EXERCISE 9.1

1. Find the complement of each of the following angles:

(a) (b) (c)
60° 82° 25°

2. Find the supplement of each of the following angles:

168°
(a) (b) (c) 5°
62°

140