Page 19 - Start Up Mathematics_8 (Non CCE)

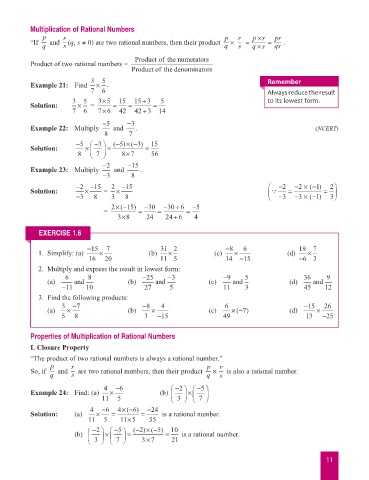
Multiplication of Rational Numbers
p r p r pr pr
×
“If q and (q, s ≠ 0) are two rational numbers, then their product q × s = qs = qs .
s
×
Productofthe numerators
Product of two rational numbers =
Productofthe denominators
3 5 Remember
Example 21: Find × .
7 6 Always reduce the result
3 5 35× 15 15 3÷ 5 to its lowest form.
Solution: × = = = =
7 6 76× 42 42 3÷ 14

−5 −3
Example 22: Multiply and . (NCERT)
8 7
−5  3 ( −× −5) ( 3) 15
− 
Solution: ×   = =
8  7  87 56
×
−2 −15
Example 23: Multiply and .
−3 8
−2 −15 2 − 15  −2 −× −1( )  2
2
Solution: × = ×   = = 
−3 8 3 8  −3 −× −1( )  3
3
2×− ( 15) − 30 − 30 6 − 5
÷
= = = =
38× 24 24 6÷ 4
EXERCISE 1.6

−15 7 31 2 −8 6 19 7
1. Simplify: (a) × (b) × (c) × (d) ×
16 20 11 5 14 −15 − 6 3
2. Multiply and express the result in lowest form:
6 8 − −25 3 −9 5 36 9
(a) and (b) and (c) and (d) and
− 11 10 27 5 11 3 45 12
3. Find the following products:
3 − 7 −8 4 6 −15 26
(a) × (b) × (c) ×−( 7) (d) ×
5 8 3 −15 49 13 −25

Properties of Multiplication of Rational Numbers
I. Closure Property

“The product of two rational numbers is always a rational number.”
p r p r
So, if q and are two rational numbers, then their product q × s is also a rational number.
s

−   5
4 − 6  2 − 
Example 24: Find: (a) × (b)    
×
11 5  3   7 
4 − 6 4×−( 6) − 24
Solution: (a) × = = is a rational number.
11 5 11 5 55
×
 2 −  ( −× −5) ( ) 10
2
−   5
×
(b)     = = is a rational number.
×
 3   7  37 21
11