Page 23 - Start Up Mathematics_8 (Non CCE)

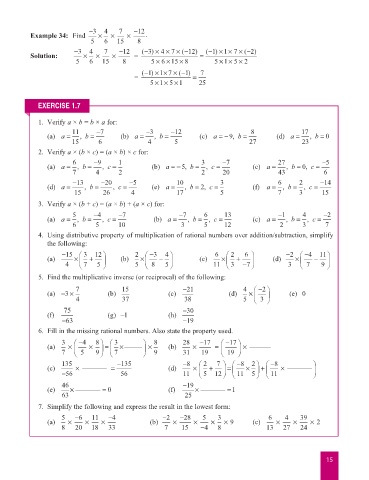
−3 4 7 −12
Example 34: Find × × × .
5 6 15 8
−3 4 7 −12 (−3 ) ×××4 7 (−12 ) ()−× ××1 1 7 (−2 )
Solution: × × × = =
5 6 15 8 56 × 51
×× ×52
××15 8
()−× ××1 1 7 ()−1 7
= =
×× ×51
51 25

EXERCISE 1.7

1. Verify a × b = b × a for:
11 −7 −3 −12 8 17
(a) a = , b = (b) a = , b = (c) a =−9, b = (d) a = , b = 0
15 6 4 5 27 23
2. Verify a × (b × c) = (a × b) × c for:

6 −9 1 3 −7 27 −5
(a) a = , b = , c = (b) a =−5, b = , c = (c) a = , b = 0, c =
7 4 2 2 20 43 6
−13 −20 −5 10 3 6 2 −14
(d) a = , b = , c = (e) a = , b = 2, c = (f) a = , b = , c =
15 26 4 17 5 7 3 15
3. Verify a × (b + c) = (a × b) + (a × c) for:
5 −4 −7 −7 6 13 −1 4 −2
(a) a = , b = , c = (b) a = , b = , c = (c) a = , b = , c =
6 5 10 3 5 12 2 3 7
4. Using distributive property of multiplication of rational numbers over addition/subtraction, simplify
the following:
−15  3 12  2  − 3 4  6  2 6  −2  −4 11 
(a) ×  +  (b) ×  −  (c) ×  +  (d) ×  − 
4  7 5  5  8 5  11  3 − 7  3  7 9 

5. Find the multiplicative inverse (or reciprocal) of the following:
7 15 −21 4  − 2 
(a) −×3 (b) (c) (d) ×   (e) 0
4 37 38 5  3 
75 −30
(f) (g) –1 (h)
− 63 −19
6. Fill in the missing rational numbers. Also state the property used.
3  − 4 8   3 _____  8 28 − 17  − 17  ______
=
(a) ×  ×   ×  × (b) × =   ×
7  5 9   7  9 31 19  19 
135 _______ − 135 −8  2 7   −8 2   −8 _______ 
=
(c) × = (d) ×  +   ×  +  × 
− 56 56 11  5 12   11 5   11 
46 _______ −19 _______
(e) × = 0 (f) × =1
63 25
7. Simplify the following and express the result in the lowest form:

5 − 6 11 − 4 −2 −28 5 3 6 4 39
(a) × × × (b) × × × × 9 (c) × × × 2
8 20 18 33 7 15 −4 8 13 27 24

15