Page 21 - Start Up Mathematics_8 (Non CCE)

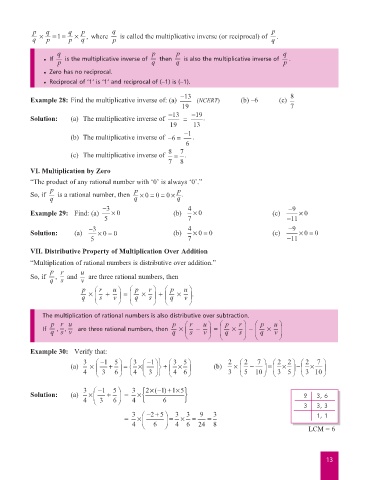
p q q p q p
1
q × p = = p × q , where p is called the multiplicative inverse (or reciprocal) of q .
q p p q
l If is the multiplicative inverse of then is also the multiplicative inverse of .
p q q p
l Zero has no reciprocal.
l Reciprocal of ‘1’ is ‘1’ and reciprocal of (–1) is (–1).

−13 8
Example 28: Find the multiplicative inverse of: (a) (NCERT) (b) –6 (c)
19 7
−13 −19
Solution: (a) The multiplicative inverse of = .
19 13
(b) The multiplicative inverse of −=6 −1 .
6
7
(c) The multiplicative inverse of 8 = .
7 8
VI. Multiplication by Zero

“The product of any rational number with ‘0’ is always ‘0’.”
p p p
So, if is a rational number, then ×= =×0 0 0 .
q q q
−3 4 −9
Example 29: Find: (a) × 0 (b) × 0 (c) × 0
5 7 −11

Solution: (a) −3 ×=0 0 (b) 4 ×= 0 (c) −9 ×=0 0
0
5 7 −11
VII. Distributive Property of Multiplication Over Addition
“Multiplication of rational numbers is distributive over addition.”
p r u
So, if , and are three rational numbers, then
q s v
p r  u   p r   p u 
q ×   s + v  =   q × s   +   q × v  .


The multiplication of rational numbers is also distributive over subtraction.
p r u p r  u   p r   p u 
,,
If q s v are three rational numbers, then q ×   s − v   =   q × s   −  q × v  

Example 30: Verify that:
3  − 1 5   3  − 1  3 5  2  2 7   2 2   2 7 
(a) ×  +  =  ×   +  ×  (b) ×  −   ×   × 
=
−
4  3 6   4  3   4 6  3  5 10   3 5   3 10 
1
()
15
3  − 1 5  3 { 2×− +× }
Solution: (a) ×  +  = × 2 3, 6
4  3 6  4 6
3 3, 3
25 
3  −+ 3 3 9 3 1, 1
= ×   = × = =
4  6  4 6 24 8
LCM = 6

13