Page 24 - Start Up Mathematics_8 (Non CCE)

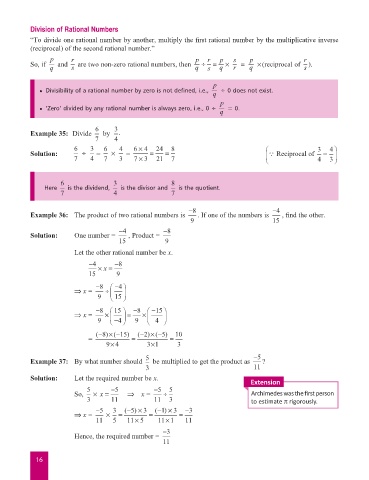
Division of Rational Numbers
“To divide one rational number by another, multiply the first rational number by the multiplicative inverse
(reciprocal) of the second rational number.”
p r p r p s p r
So, if q and are two non-zero rational numbers, then q ÷ s = q × r = q ×(reciprocal of ).
s
s
p
l Divisibility of a rational number by zero is not defined, i.e., q ÷ 0 does not exist.
p
l ‘Zero’ divided by any rational number is always zero, i.e., 0 ÷ q = 0.

6 3
Example 35: Divide by .
7 4
6 3 6 4 64× 24 8  3 4 
Solution: ∏ = × = = =   Reciprocal of = 
7 4 7 3 73× 21 7  4 3 

6 3 8
Here is the dividend, is the divisor and is the quotient.
7 4 7

−8 −4
Example 36: The product of two rational numbers is . If one of the numbers is , find the other.
9 15

−4 −8
Solution: One number = , Product =
15 9
Let the other rational number be x.
−4 −8
×=
x
15 9
−8  4
− 
⇒ x = ÷  
9  15 
−8  15  −8  −15 
⇒ x = ×   = ×  
9  4 9  4 
− 
) (−15
2
8
(−× ) (−× ) 10
) (−5
= = =
94 31 3
×
×
5
Example 37: By what number should be multiplied to get the product as −5 ?
3 11
Solution: Let the required number be x. Extension
5 − 5 −5 5
So, × x = ⇒ x = ÷ Archimedes was the first person
3 11 11 3 to estimate π rigorously.
−5 3 ( −5) × 3 () 3 −3
−×1
⇒ x = × = = =
11 5 11 × 5 11 ×1 11
−3
Hence, the required number =
11

16