Page 28 - Start Up Mathematics_8 (Non CCE)

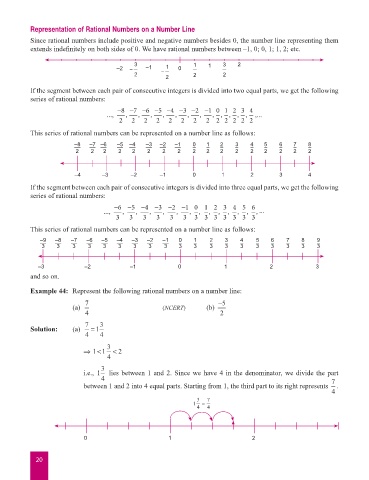
Representation of Rational Numbers on a Number Line

Since rational numbers include positive and negative numbers besides 0, the number line representing them
extends indefi nitely on both sides of 0. We have rational numbers between –1, 0; 0, 1; 1, 2; etc.

3 1 1 3 2
–2 − –1 1 0
2 − 2 2 2

If the segment between each pair of consecutive integers is divided into two equal parts, we get the following
series of rational numbers:
− −8 −7 −6 −5 −4 −3 −2 1 0 1 2 3 4
..., , , , , , , , ,,,,, ,...
2 2 2 2 2 2 2 2 2 2 2 2 2

This series of rational numbers can be represented on a number line as follows:
–8 –7 –6 –5 –4 –3 –2 –1 0 1 2 3 4 5 6 7 8
2 2 2 2 2 2 2 2 2 2 2 2 2 2 2 2 2

–4 –3 –2 –1 0 1 2 3 4

If the segment between each pair of consecutive integers is divided into three equal parts, we get the following
series of rational numbers:

− −6 5 − 3 − 2 −4 − 1 0 1 2 3 4 5 6
..., , , , , , , , , , , , , , ...
3 3 3 3 3 3 3 3 3 3 3 3 3
This series of rational numbers can be represented on a number line as follows:

–9 –8 –7 –6 –5 –4 –3 –2 –1 0 1 2 3 4 5 6 7 8 9
3 3 3 3 3 3 3 3 3 3 3 3 3 3 3 3 3 3 3

–3 –2 –1 0 1 2 3
and so on.

Example 44: Represent the following rational numbers on a number line:
7 −5
(a) (NCERT) (b)
4 2

7 3
Solution: (a) = 1
4 4
3
⇒ 11< < 2
4

3
i.e., 1 lies between 1 and 2. Since we have 4 in the denominator, we divide the part
4 7
between 1 and 2 into 4 equal parts. Starting from 1, the third part to its right represents .
4
3 7
1 =
4 4

0 1 2

20