Page 27 - Start Up Mathematics_8 (Non CCE)

3. Show that a ÷ (b ÷ c) ≠ (a ÷ b) ÷ c, for:
3 −7 −2 1 −1 −4 −12 −3
(a) a = , b = , c = 5 (b) a = , b = , c = (c) a = , b = , c =
2 6 3 2 7 9 20 5
33 9
4. The product of two rational numbers is . If one of the numbers is , find the other number.
52 13

5
5. The product of two rational numbers is –20. If one of the numbers is , find the other number.
6
−5 7
6. By what number should be multiplied to get the product as ?
14 12
28 −7
7. By what number should we multiply to get the product as ?
− 15 5
−12 3
8. By what number should be divided to get ?
35 7
−12 13 −1 31
9. Divide the sum of and by the product of and .
7 5 2 7
8 12
10. Divide the sum of and by their difference.
3 7

MATHS LAB ACTIVITY
Objective: To learn about rational numbers and its various operations pictorically

Material required: Art sheet, coloured papers, sketch pens, glue, protractor, compass, scissors
2 3
Step 1: Choose two rational numbers. For example, and
9 5
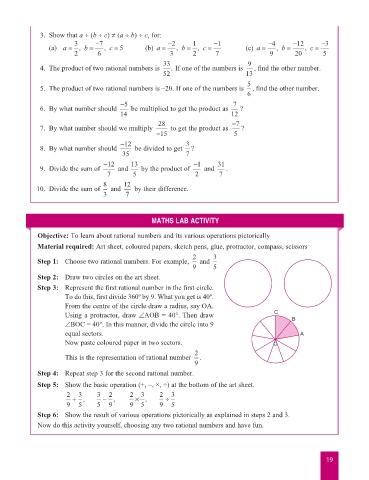
Step 2: Draw two circles on the art sheet.
Step 3: Represent the first rational number in the first circle.
To do this, first divide 360º by 9. What you get is 40º.
From the centre of the circle draw a radius, say OA.
Using a protractor, draw ∠AOB = 40°. Then draw C B
∠BOC = 40°. In this manner, divide the circle into 9
equal sectors. A
Now paste coloured paper in two sectors. O
2
This is the representation of rational number .
9

Step 4: Repeat step 3 for the second rational number.
Step 5: Show the basic operation (+, –, ×, ÷) at the bottom of the art sheet.
2 3 3 2 2 3 2 3
+ , − , × , ÷
9 5 5 9 9 5 9 5
Step 6: Show the result of various operations pictorically as explained in steps 2 and 3.
Now do this activity yourself, choosing any two rational numbers and have fun.

19