Page 17 - Start Up Mathematics_8 (Non CCE)

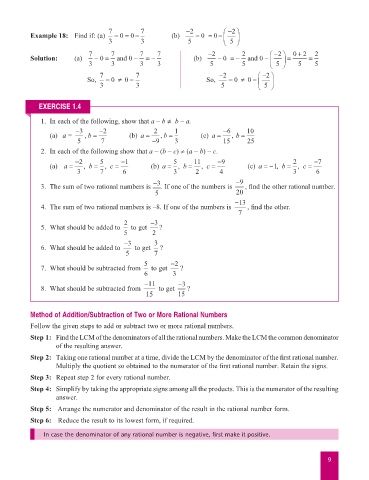
7 7 −2  2
− 
0
0
Example 18: Find if: (a) −= 0 − (b) − 0 = −  
3 3 5  5 
7 7 7 7 −2 2  2 02 2
+
− 
0
Solution: (a) −= and 0 − = − (b) − 0 = − and 0 −   = =
3 3 3 3 5 5  5  5 5
7 7 −2  − 2 
So, − 0 ≠ 0 − So, − 0 ≠ 0 −  
3 3 5  5 
EXERCISE 1.4

1. In each of the following, show that a – b ≠ b – a.
−3 −2 2 1 −6 10
(a) a = , b = (b) a = , b = (c) a = , b =
5 7 −9 3 15 25
2. In each of the following show that a – (b – c) ≠ (a – b) – c.
−2 5 −1 5 11 −9 2 −7
(a) a = , b = , c = (b) a = , b = , c = (c) a =−1, b = , c =
3 7 6 3 2 4 3 6

3. The sum of two rational numbers is −3 . If one of the numbers is −9 , find the other rational number.
5 20
−13
4. The sum of two rational numbers is –8. If one of the numbers is , find the other.
7
2 −3
5. What should be added to to get ?
5 2
−3 3
6. What should be added to to get ?
5 7
5 −2
7. What should be subtracted from to get ?
6 3
−11 −3
8. What should be subtracted from to get ?
15 15

Method of Addition/Subtraction of Two or More Rational Numbers
Follow the given steps to add or subtract two or more rational numbers.
Step 1: Find the LCM of the denominators of all the rational numbers. Make the LCM the common denominator
of the resulting answer.
Step 2: Taking one rational number at a time, divide the LCM by the denominator of the first rational number.
Multiply the quotient so obtained to the numerator of the first rational number. Retain the signs.
Step 3: Repeat step 2 for every rational number.

Step 4: Simplify by taking the appropriate signs among all the products. This is the numerator of the resulting
answer.
Step 5: Arrange the numerator and denominator of the result in the rational number form.
Step 6: Reduce the result to its lowest form, if required.

In case the denominator of any rational number is negative, first make it positive.

9