Page 14 - Start Up Mathematics_8 (Non CCE)

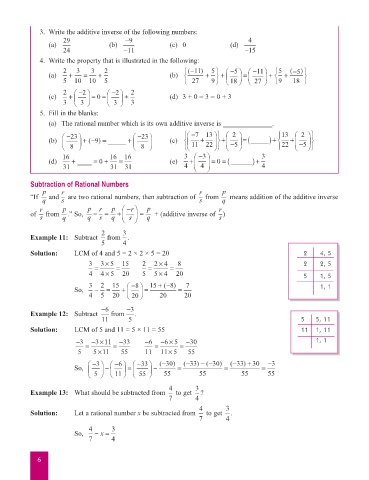
3. Write the additive inverse of the following numbers:
29 −9 4
(a) (b) (c) 0 (d)
24 −11 − 15
4. Write the property that is illustrated in the following:
2 3 3 2  (−11 ) 5   5 −11  5 − ( 5 )
−  
=
(a) + = + (b)  +  +     +  + 
5 10 10 5  27 9   18   27  9 18 
2  − 2   − 2  2
(c) +   ==   + (d) 3 + 0 = 3 = 0 + 3
0
3  3   3  3
5. Fill in the blanks:
(a) The rational number which is its own additive inverse is ______________.
 −23   −23    −7 13   2  13  2 
(b)   +−9( ) = _____ +   (c)   +  +   =(_____ )+  +  
 8   8    11 22   −5   22  −5 
16 16 16 3  − 3  3
0 (______
(d) + ____ = 0 + = (e) +   == ) +
31 31 31 4  4  4

Subtraction of Rational Numbers
p r r p
“If q and are two rational numbers, then subtraction of from q means addition of the additive inverse
s
s
r p p r p  r −  p r
of from .” So, q − s = q +   s   = q + (additive inverse of )
s
q
s
2 3
Example 11: Subtract from .
5 4
Solution: LCM of 4 and 5 = 2 × 2 × 5 = 20 2 4, 5
3 = 35× = 15 2 = 24× = 8 2 2, 5
4 45× 20 5 54× 20 5 1, 5

3 2 15  − 8  15 +−( 8) 7 1, 1
So, − = +   = =
4 5 20  20  20 20

− −6 3
Example 12: Subtract from .
11 5 5 5, 11
Solution: LCM of 5 and 11 = 5 × 11 = 55 11 1, 11
−3 −×311 −33 −6 −×65 −30 1, 1
= = = =
×
5 511 55 11 11 × 5 55
 3 −   −33  ( −30) ( −33) − −30( ) ( −333) + 30 − 3
−   6
So,       − = = =
=
−
 5   11   55  55 55 55 55
4 3
Example 13: What should be subtracted from to get ?
7 4
4 3
Solution: Let a rational number x be subtracted from to get .
7 4
4 3
x
So, −=
7 4

6