Page 10 - Start Up Mathematics_8 (Non CCE)

− −4 −5 −7 2
Example 1: Compare , , , .
9 12 18 3 3 9, 12,18, 3
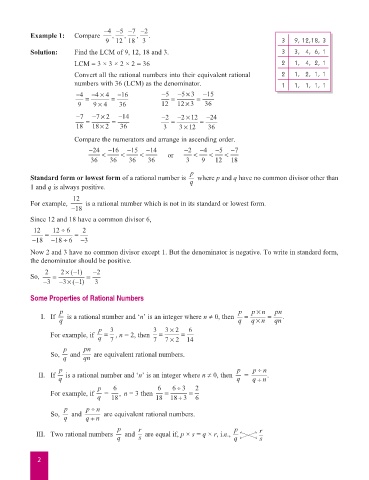
Solution: Find the LCM of 9, 12, 18 and 3. 3 3, 4, 6, 1
LCM = 3 × 3 × 2 × 2 = 36 2 1, 4, 2, 1

Convert all the rational numbers into their equivalent rational 2 1, 2, 1, 1
numbers with 36 (LCM) as the denominator. 1 1, 1, 1, 1

−4 = −×4 4 = −16 −5 = −×53 = −15
9 9 × 4 36 12 12 × 3 36
−7 −×72 −14 −2 −×212 −24
18 = 18 × 2 = 36 3 = 312 = 36
×
Compare the numerators and arrange in ascending order.
−24 < −16 < −15 < −14 or −2 < −4 < −5 < −7
36 36 36 36 3 9 12 18

p
Standard form or lowest form of a rational number is q where p and q have no common divisor other than
1 and q is always positive.

12
For example, is a rational number which is not in its standard or lowest form.
− 18
Since 12 and 18 have a common divisor 6,
12 = 12 6÷ = 2
− 18 − 18 6÷ − 3

Now 2 and 3 have no common divisor except 1. But the denominator is negative. To write in standard form,
the denominator should be positive.
2 2 ×−( 1) − 2
So, = =
3
− 3 −× −( 1) 3
Some Properties of Rational Numbers

×
p p pn pn
I. If q is a rational number and ‘n’ is an integer where n ≠ 0, then q = qn× = qn .
p 3 3 3 × 2 6
For example, if q = 7 , n = 2, then 7 = 7 × 2 = 14

p pn
So, and are equivalent rational numbers.
q qn
∏
p p pn
II. If is a rational number and ‘n’ is an integer where n ≠ 0, then q = qn .
q
∏
p 6 6 63÷ 2
For example, if = 18 , n = 3 then 18 = 18 3÷ = 6
q
p pn
∏
So, q and qn are equivalent rational numbers.
∏
p r p
III. Two rational numbers q and are equal if, p × s = q × r, i.e., q ←→ r s
s
←→

2