Page 16 - Start Up Mathematics_8 (Non CCE)

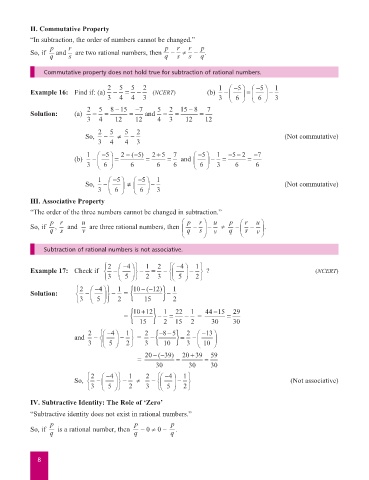
II. Commutative Property
“In subtraction, the order of numbers cannot be changed.”
p r p r r p
So, if q and s are two rational numbers, then q − ≠ − q .
s
s
Commutative property does not hold true for subtraction of rational numbers.

2 5 5 2 1  − 5   − 5  1
Example 16: Find if: (a) − = − (NCERT) (b) −     −
=
3 4 4 3 3  6   6  3
2 5 815− − 7 5 2 15 8− 7
Solution: (a) − = = and − = =
3 4 12 12 4 3 12 12
2 5 5 2
So, − ≠ − (Not commutative)
3 4 4 3

1  − 5  2 −−( 5) 25+ 7  5 1 −−52 −7
− 
(b) −   = = = and   − = =
3  6  6 6 6  6  3 6 6
1  − 5   5 1
− 
So, −   ≠   − (Not commutative)
3  6   6  3
III. Associative Property

“The order of the three numbers cannot be changed in subtraction.”
p r u  p r  u p r  u 
So, if , and are three rational numbers, then  −  − ≠ −  −  .
q s v  q s  v q  s v 

Subtraction of rational numbers is not associative.

 2  − 4  1 2   − 4  1
Example 17: Check if  −   − = −    −  ? (NCERT)
 3  5  2 3   5  2 
 2  − 4  1 { 10 −− ( 12) } 1
Solution:  −   − = −
 3  5  2 15 2
{ 10 12+ } 1 22 1 44 15− 29
= 15 − 2 = 15 − 2 = 30 = 30

−−
2   − 4  1 2 { } 2  − 13 
85
and −    −  = − = −  
3   5  2  3 10 3  10 
20 −−( 39) 20 39+ 59
= = =
30 30 30
 2  − 4  1 2   − 4  1
So,  −   − ≠ −    −  (Not associative)
 3  5  2 3   5  2 
IV. Subtractive Identity: The Role of ‘Zero’

“Subtractive identity does not exist in rational numbers.”
p p p
So, if q is a rational number, then q – 0 ≠ 0 – q .

8