Page 191 - Start Up Mathematics_7

∆ DEF ∆ PQR

(a) ∠D = 60°, ∠F = 80°, DF = 6 cm ∠Q = 60°, ∠R = 80°, QR = 6 cm
(b) ∠E = 80°, ∠F = 30°, EF = 5 cm ∠P = 80°, PQ = 5 cm, ∠R = 40°

Solution: (a) ∆ DEF ≅ ∆ PQR. By angle sum property in ∆ PQR we find ∠P and show ∠D ≠ ∠P.
In fact the correct matching of vertices by ASA rule gives the correspondence
DEF ↔ QPR, hence ∆ DEF  ∆ QPR.

(b) ∆ DEF ≅ ∆ PQR as ∠F ≠ ∠R. In fact the triangles are not congruent under any
correspondence.
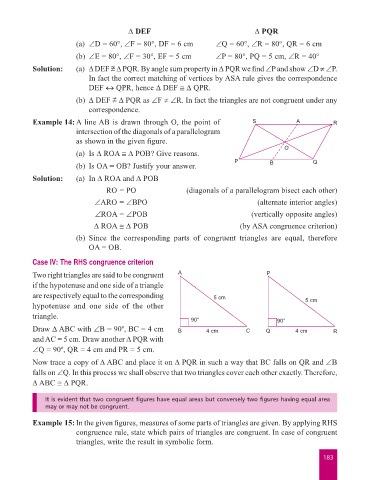
Example 14: A line AB is drawn through O, the point of S A R
intersection of the diagonals of a parallelogram
as shown in the given figure.
O
(a) Is ∆ ROA  ∆ POB? Give reasons.
P B Q
(b) Is OA = OB? Justify your answer.

Solution: (a) In ∆ ROA and ∆ POB
RO = PO (diagonals of a parallelogram bisect each other)

∠ARO = ∠BPO (alternate interior angles)
∠ROA = ∠POB (vertically opposite angles)

∆ ROA  ∆ POB (by ASA congruence criterion)
(b) Since the corresponding parts of congruent triangles are equal, therefore
OA = OB.

Case IV: The RHS congruence criterion

Two right triangles are said to be congruent A P
if the hypotenuse and one side of a triangle
are respectively equal to the corresponding 5 cm 5 cm
hypotenuse and one side of the other
triangle.
90° 90°
Draw ∆ ABC with ∠B = 90º, BC = 4 cm B 4 cm C Q 4 cm R
and AC = 5 cm. Draw another ∆ PQR with
∠Q = 90º, QR = 4 cm and PR = 5 cm.

Now trace a copy of ∆ ABC and place it on ∆ PQR in such a way that BC falls on QR and ∠B
falls on ∠Q. In this process we shall observe that two triangles cover each other exactly. Therefore,
∆ ABC  ∆ PQR.

It is evident that two congruent figures have equal areas but conversely two figures having equal area
may or may not be congruent.

Example 15: In the given figures, measures of some parts of triangles are given. By applying RHS
congruence rule, state which pairs of triangles are congruent. In case of congruent
triangles, write the result in symbolic form.

183