Page 189 - Start Up Mathematics_7

Solution: (a) In ∆ DFE and ∆ PQR

DF = PQ (each 4 cm)
∠ F = ∠Q (each 40°)

FE = QR (each 3 cm)

∴ ∆ DFE  ∆ PQR (by SAS congruence criterion)
(b) In ∆ QPR  ∆ SRP

QP = SR (each 3.8 cm)
∠QPR = ∠SRP (each 40°)

PR = RP (common)
∴ ∆ QPR  ∆ SRP (by SAS congruence criterion)

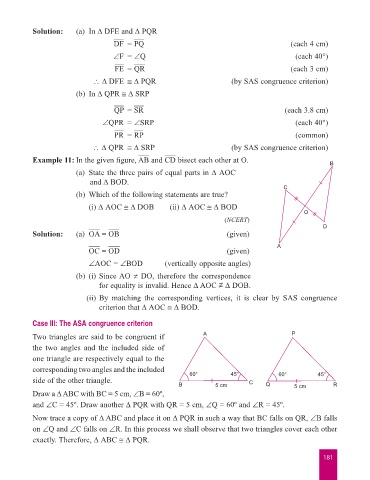
Example 11: In the given figure, AB and CD bisect each other at O.
B
(a) State the three pairs of equal parts in ∆ AOC
and ∆ BOD.
C
(b) Which of the following statements are true?

(i) ∆ AOC  ∆ DOB (ii) ∆ AOC  ∆ BOD
O
(NCERT)
D
Solution: (a) OA = OB (given)

A
OC = OD (given)
∠AOC = ∠BOD (vertically opposite angles)

(b) (i) Since AO ≠ DO, therefore the correspondence
for equality is invalid. Hence ∆ AOC ≅ ∆ DOB.
(ii) By matching the corresponding vertices, it is clear by SAS congruence
criterion that ∆ AOC  ∆ BOD.

Case III: The ASA congruence criterion
Two triangles are said to be congruent if A P

the two angles and the included side of
one triangle are respectively equal to the
corresponding two angles and the included 60° 45° 60° 45°
side of the other triangle. C
B 5 cm Q 5 cm R
Draw a ∆ ABC with BC = 5 cm, ∠B = 60º,
and ∠C = 45º. Draw another ∆ PQR with QR = 5 cm, ∠Q = 60º and ∠R = 45º.

Now trace a copy of ∆ ABC and place it on ∆ PQR in such a way that BC falls on QR, ∠B falls
on ∠Q and ∠C falls on ∠R. In this process we shall observe that two triangles cover each other

exactly. Therefore, ∆ ABC  ∆ PQR.

181