Page 185 - Start Up Mathematics_7

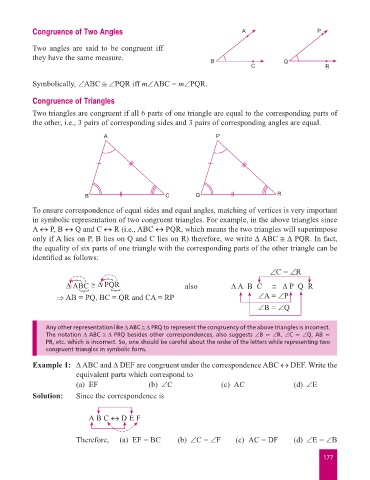
Congruence of Two Angles A P

Two angles are said to be congruent iff
they have the same measure.
B Q
C R

Symbolically, ∠ABC  ∠PQR iff m∠ABC = m∠PQR.

Congruence of Triangles
Two triangles are congruent if all 6 parts of one triangle are equal to the corresponding parts of
the other, i.e., 3 pairs of corresponding sides and 3 pairs of corresponding angles are equal.

A P

B C Q R
To ensure correspondence of equal sides and equal angles, matching of vertices is very important
in symbolic representation of two congruent triangles. For example, in the above triangles since
A ↔ P, B ↔ Q and C ↔ R (i.e., ABC ↔ PQR, which means the two triangles will superimpose
only if A lies on P, B lies on Q and C lies on R) therefore, we write ∆ ABC ≅ ∆ PQR. In fact,
the equality of six parts of one triangle with the corresponding parts of the other triangle can be
identified as follows:

∠C = ∠R

∆ ABC ≅ ∆ PQR also ∆ A B C ≅ ∆ P Q R
⇒ AB = PQ, BC = QR and CA = RP ∠A = ∠P
∠B = ∠Q

Any other representation like ∆ ABC ≅ ∆ PRQ to represent the congruency of the above triangles is incorrect.
The notation ∆ ABC ≅ ∆ PRQ besides other correspondences, also suggests ∠B = ∠R, ∠C = ∠Q, AB =
PR, etc. which is incorrect. So, one should be careful about the order of the letters while representing two
congruent triangles in symbolic form.

Example 1: ∆ ABC and ∆ DEF are congruent under the correspondence ABC ↔ DEF. Write the
equivalent parts which correspond to
(a) EF (b) ∠C (c) AC (d) ∠E
Solution: Since the correspondence is

A B C ↔ D E F

Therefore, (a) EF = BC (b) ∠C = ∠F (c) AC = DF (d) ∠E = ∠B

177177