Page 186 - Start Up Mathematics_7

Example 2: Complete the following statements:
(a) If two plane figures cover each other exactly, they are ______________.
(b) When we write ∠A ≅ ∠B, we actually mean ______________.
(c) If under a given correspondence, two triangles are congruent, then their
corresponding parts that match each other are ______________.
Solution: (a) congruent by superposition (b) m∠A = m∠B (c) equal
Example 3: State True or False.
(a) Two circles with equal radii are congruent.
(b) Two squares are congruent if a side of one square is equal to a side of the other.
(c) Two rhombuses are congruent if a side of one rhombus is equal to a side of the
other.
(d) Two rectangles having same lengths and breadths may not be congruent.
Solution: (a) True (b) True (c) False (d) False
Example 4: Give any three real-life examples of congruent shapes.
Solution: The three real life examples of congruent shapes are:
(a) Two one rupee coins (b) Two postcards
(c) Maps of a country drawn on the same scale
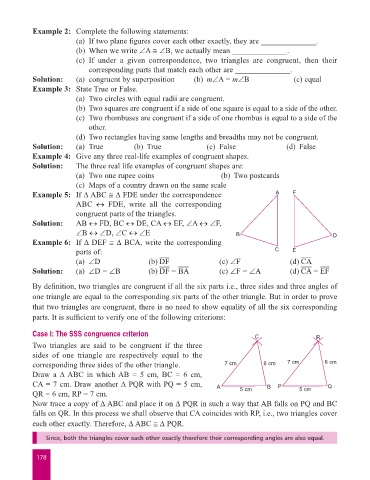
Example 5: If ∆ ABC  ∆ FDE under the correspondence A F
ABC ↔ FDE, write all the corresponding
congruent parts of the triangles.
Solution: AB ↔ FD, BC ↔ DE, CA ↔ EF, ∠A ↔ ∠F,
∠B ↔ ∠D, ∠C ↔ ∠E B D
Example 6: If ∆ DEF  ∆ BCA, write the corresponding
parts of: C E
(a) ∠D (b) DF (c) ∠F (d) CA
Solution: (a) ∠D = ∠B (b) DF = BA (c) ∠F = ∠A (d) CA = EF

By definition, two triangles are congruent if all the six parts i.e., three sides and three angles of
one triangle are equal to the corresponding six parts of the other triangle. But in order to prove
that two triangles are congruent, there is no need to show equality of all the six corresponding
parts. It is sufficient to verify one of the following criterions:

Case I: The SSS congruence criterion C R
Two triangles are said to be congruent if the three
sides of one triangle are respectively equal to the
corresponding three sides of the other triangle. 7 cm 6 cm 7 cm 6 cm
Draw a ∆ ABC in which AB = 5 cm, BC = 6 cm,
CA = 7 cm. Draw another ∆ PQR with PQ = 5 cm, A B P Q
QR = 6 cm, RP = 7 cm. 5 cm 5 cm
Now trace a copy of ∆ ABC and place it on ∆ PQR in such a way that AB falls on PQ and BC
falls on QR. In this process we shall observe that CA coincides with RP, i.e., two triangles cover
each other exactly. Therefore, ∆ ABC  ∆ PQR.

Since, both the triangles cover each other exactly therefore their corresponding angles are also equal.

178