Page 188 - Start Up Mathematics_7

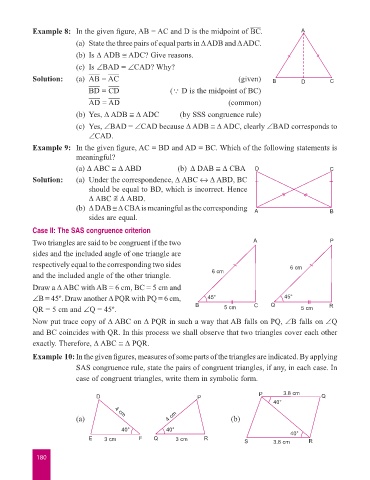
Example 8: In the given figure, AB = AC and D is the midpoint of BC. A

(a) State the three pairs of equal parts in ∆ ADB and ∆ ADC.
(b) Is ∆ ADB  ADC? Give reasons.

(c) Is ∠BAD = ∠CAD? Why?
Solution: (a) AB = AC (given) B D C

BD = CD ( D is the midpoint of BC)
AD = AD (common)
(b) Yes, ∆ ADB  ∆ ADC (by SSS congruence rule)

(c) Yes, ∠BAD = ∠CAD because ∆ ADB  ∆ ADC, clearly ∠BAD corresponds to
∠CAD.
Example 9: In the given figure, AC = BD and AD = BC. Which of the following statements is
meaningful?

(a) ∆ ABC  ∆ ABD (b) ∆ DAB  ∆ CBA D C
Solution: (a) Under the correspondence, ∆ ABC  ∆ ABD, BC
should be equal to BD, which is incorrect. Hence
∆ ABC ≅∆ABD.
(b) ∆ DAB  ∆ CBA is meaningful as the corresponding A B
sides are equal.
Case II: The SAS congruence criterion

Two triangles are said to be congruent if the two A P
sides and the included angle of one triangle are

respectively equal to the corresponding two sides 6 cm
and the included angle of the other triangle. 6 cm
Draw a ∆ ABC with AB = 6 cm, BC = 5 cm and
∠B = 45º. Draw another ∆ PQR with PQ = 6 cm, 45° 45°

QR = 5 cm and ∠Q = 45º. B 5 cm C Q 5 cm R
Now put trace copy of ∆ ABC on ∆ PQR in such a way that AB falls on PQ, ∠B falls on ∠Q

and BC coincides with QR. In this process we shall observe that two triangles cover each other
exactly. Therefore, ∆ ABC  ∆ PQR.
Example 10: In the given figures, measures of some parts of the triangles are indicated. By applying

SAS congruence rule, state the pairs of congruent triangles, if any, in each case. In
case of congruent triangles, write them in symbolic form.

D P P 3.8 cm Q
40°

(a) 4 cm 4 cm (b)

40° 40° 40°
E 3 cm F Q 3 cm R S 3.8 cm R

180