Page 193 - Start Up Mathematics_7

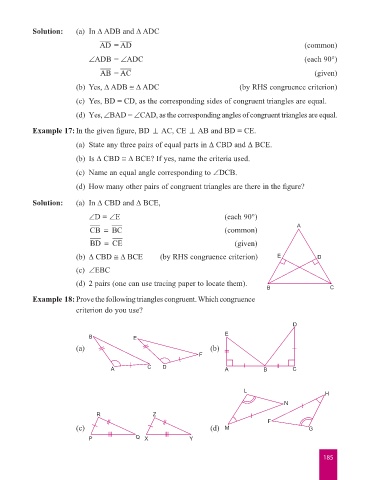
Solution: (a) In ∆ ADB and ∆ ADC

AD = AD (common)

∠ ADB = ∠ADC (each 90°)

AB = AC (given)

(b) Yes, ∆ ADB  ∆ ADC (by RHS congruence criterion)

(c) Yes, BD = CD, as the corresponding sides of congruent triangles are equal.

(d) Yes, ∠BAD = ∠CAD, as the corresponding angles of congruent triangles are equal.

Example 17: In the given figure, BD AC, CE AB and BD = CE.

(a) State any three pairs of equal parts in ∆ CBD and ∆ BCE.

(b) Is ∆ CBD  ∆ BCE? If yes, name the criteria used.
(c) Name an equal angle corresponding to ∠DCB.

(d) How many other pairs of congruent triangles are there in the figure?

Solution: (a) In ∆ CBD and ∆ BCE,

∠D = ∠E (each 90°)
A
(common)
(given)

(b) ∆ CBD  ∆ BCE (by RHS congruence criterion) E D

(c) ∠EBC
(d) 2 pairs (one can use tracing paper to locate them).
B C
Example 18: Prove the following triangles congruent. Which congruence
criterion do you use?

D
E
B E
(a) (b)
F

A C D A B C

L H
N

R Z
F
(c) (d) M G

P Q X Y

185