Page 194 - Start Up Mathematics_7

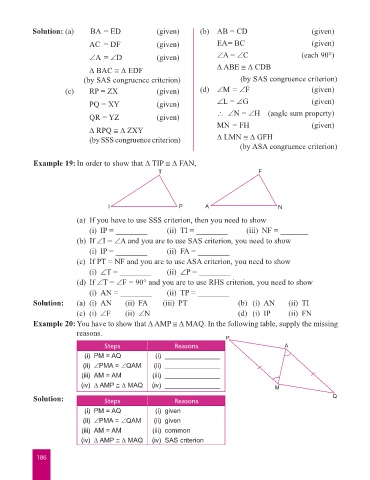
Solution: (a) BA = ED (given) (b) AB = CD (given)

AC = DF (given) EA = BC (given)

∠A = ∠D (given) ∠A = ∠C (each 90°)

∆ BAC  ∆ EDF ∆ ABE  ∆ CDB
(by SAS congruence criterion) (by SAS congruence criterion)

(c) RP = ZX (given) (d) ∠M = ∠F (given)
PQ = XY (given) ∠L = ∠G (given)
∴ ∠N = ∠H (angle sum property)
QR = YZ (given)
MN = FH (given)
∆ RPQ  ∆ ZXY
(by SSS congruence criterion) ∆ LMN  ∆ GFH
(by ASA congruence criterion)

Example 19: In order to show that ∆ TIP  ∆ FAN,

T F

I P A N

(a) If you have to use SSS criterion, then you need to show
(i) IP = ________ (ii) TI = ________ (iii) NF = _______
(b) If ∠I = ∠A and you are to use SAS criterion, you need to show

(i) IP = ________ (ii) FA = ________
(c) If PT = NF and you are to use ASA criterion, you need to show
(i) ∠T = ________ (ii) ∠P = ________
(d) If ∠T = ∠F = 90° and you are to use RHS criterion, you need to show
(i) AN = ________ (ii) TP = ________
Solution: (a) (i) AN (ii) FA (iii) PT (b) (i) AN (ii) TI

(c) (i) ∠F (ii) ∠N (d) (i) IP (ii) FN
Example 20: You have to show that ∆ AMP  ∆ MAQ. In the following table, supply the missing
reasons.
P
Steps Reasons A
(i) PM = AQ (i) _______________
(ii) ∠PMA = ∠QAM (ii) _______________
(iii) AM = AM (iii) _______________
(iv) ∆ AMP ≅ ∆ MAQ (iv) _______________ M

Solution: Steps Reasons Q

(i) PM = AQ (i) given
(ii) ∠PMA = ∠QAM (ii) given
(iii) AM = AM (iii) common
(iv) ∆ AMP ≅ ∆ MAQ (iv) SAS criterion

186