Page 196 - Start Up Mathematics_7

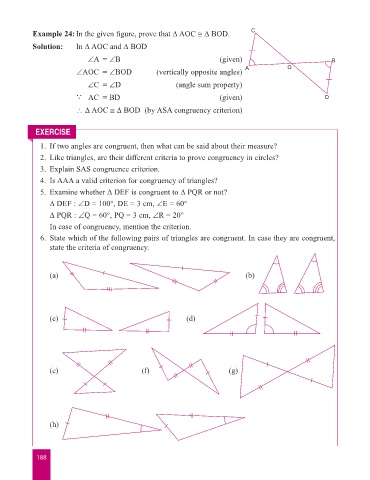
Example 24: In the given figure, prove that ∆ AOC  ∆ BOD. C
Solution: In ∆ AOC and ∆ BOD

∠A = ∠B (given) B

∠AOC = ∠BOD (vertically opposite angles) A O
∠C = ∠D (angle sum property)

∵ AC = BD (given) D

∴ ∆ AOC  ∆ BOD (by ASA congruency criterion)

EXERCISE

1. If two angles are congruent, then what can be said about their measure?
2. Like triangles, are their different criteria to prove congruency in circles?

3. Explain SAS congruence criterion.
4. Is AAA a valid criterion for congruency of triangles?
5. Examine whether ∆ DEF is congruent to ∆ PQR or not?

∆ DEF : ∠D = 100°, DE = 3 cm, ∠E = 60°
∆ PQR : ∠Q = 60°, PQ = 3 cm, ∠R = 20°

In case of congruency, mention the criterion.
6. State which of the following pairs of triangles are congruent. In case they are congruent,
state the criteria of congruency.

(a) (b)

(c) (d)

(e) (f) (g)

(h)

188