Page 201 - Start Up Mathematics_7

4. In two congruent triangles, how can you determine corresponding equal sides from corresponding

equal angles and vice versa?
5. What measure is sufficient to prove that 2 squares are congruent?

6. What measure is sufficient to prove the congruence of 2 circles?

let’s evaluate
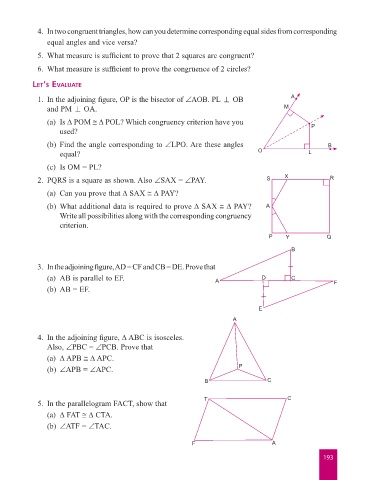
1. In the adjoining figure, OP is the bisector of ∠AOB. PL OB A
and PM OA. M

(a) Is ∆ POM ≅ ∆ POL? Which congruency criterion have you P
used?

(b) Find the angle corresponding to ∠LPO. Are these angles B
equal? O L
(c) Is OM = PL?

2. PQRS is a square as shown. Also ∠SAX = ∠PAY. S X R

(a) Can you prove that ∆ SAX ≅ ∆ PAY?

(b) What additional data is required to prove ∆ SAX ≅ ∆ PAY? A
Write all possibilities along with the corresponding congruency
criterion.

P Y Q

B

3. In the adjoining figure, AD = CF and CB = DE. Prove that
(a) AB is parallel to EF. A D C F
(b) AB = EF.

E
A

4. In the adjoining figure, ∆ ABC is isosceles.
Also, ∠PBC = ∠PCB. Prove that

(a) ∆ APB ≅ ∆ APC.
(b) ∠APB = ∠APC. P

B C

T C
5. In the parallelogram FACT, show that
(a) ∆ FAT ≅ ∆ CTA.

(b) ∠ATF = ∠TAC.

F A

193