Page 205 - Start Up Mathematics_7

12 Constructions

Euclid, a Greek mathematician mentioned construction techniques in his
book titled Elements. These techniques have become a very important part
of geometry and are taught even today. He explained that construction can

be done by using only a compass and a ruler without measuring the lengths
or angles.

In the previous class, we have learnt the construction of a circle, line segment,
perpendicular lines, perpendicular bisector of a line segment, special angles
and angle bisectors using a ruler and compass. In this lesson, we shall learn how to draw parallel
lines and triangles using a ruler and compass.

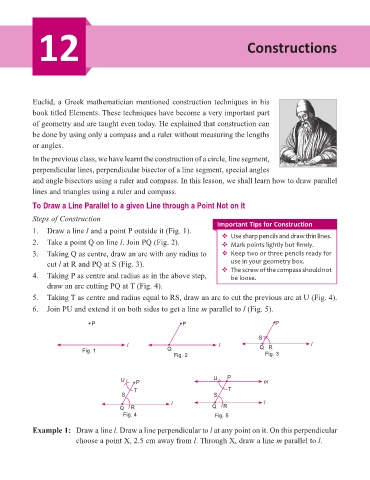
To Draw a Line Parallel to a given Line through a Point Not on It

Steps of Construction Important Tips for Construction
1. Draw a line l and a point P outside it (Fig. 1).

2. Take a point Q on line l. Join PQ (Fig. 2).  Use sharp pencils and draw thin lines.
 Mark points lightly but firmly.
3. Taking Q as centre, draw an arc with any radius to  Keep two or three pencils ready for
cut l at R and PQ at S (Fig. 3). use in your geometry box.

4. Taking P as centre and radius as in the above step,  The screw of the compass should not
be loose.
draw an arc cutting PQ at T (Fig. 4).
5. Taking T as centre and radius equal to RS, draw an arc to cut the previous arc at U (Fig. 4).

6. Join PU and extend it on both sides to get a line m parallel to l (Fig. 5).

P P P

S
l l Q R l
Fig. 1 Q
Fig. 2 Fig. 3

U P U P m
T T
S S
l l
Q R Q R
Fig. 4 Fig. 5

Example 1: Draw a line l. Draw a line perpendicular to l at any point on it. On this perpendicular
choose a point X, 2.5 cm away from l. Through X, draw a line m parallel to l.