Page 256 - Start Up Mathematics_8 (Non CCE)

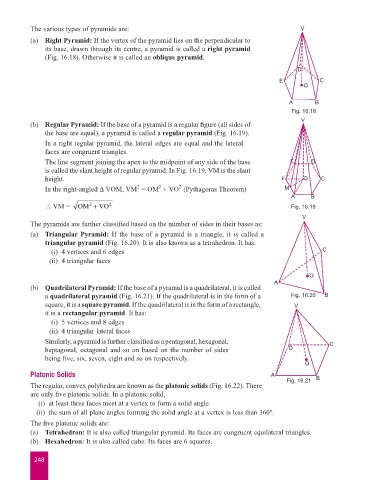
The various types of pyramids are: V

(a) Right Pyramid: If the vertex of the pyramid lies on the perpendicular to
its base, drawn through its centre, a pyramid is called a right pyramid
(Fig. 16.18). Otherwise it is called an oblique pyramid.

D
E C
O

A B
Fig. 16.18
V
(b) Regular Pyramid: If the base of a pyramid is a regular figure (all sides of
the base are equal), a pyramid is called a regular pyramid (Fig. 16.19).
In a right regular pyramid, the lateral edges are equal and the lateral
faces are congruent triangles.
The line segment joining the apex to the midpoint of any side of the base E D
is called the slant height of regular pyramid. In Fig. 16.19, VM is the slant
height. F O C
2
2
2
In the right-angled D VOM, VM = OM + VO (Pythagoras Theorem) M
A B
2
\ VM = OM + VO 2 Fig. 16.19
V
The pyramids are further classified based on the number of sides in their bases as:
(a) Triangular Pyramid: If the base of a pyramid is a triangle, it is called a
triangular pyramid (Fig. 16.20). It is also known as a tetrahedron. It has:
(i) 4 vertices and 6 edges C
(ii) 4 triangular faces

O
A
(b) Quadrilateral Pyramid: If the base of a pyramid is a quadrilateral, it is called
a quadrilateral pyramid (Fig. 16.21). If the quadrilateral is in the form of a Fig. 16.20 B
square, it is a square pyramid. If the quadrilateral is in the form of a rectangle, V
it is a rectangular pyramid. It has:
(i) 5 vertices and 8 edges
(ii) 4 triangular lateral faces
Similarly, a pyramid is further classified as a pentagonal, hexagonal, C
heptagonal, octagonal and so on based on the number of sides D
being five, six, seven, eight and so on respectively.
O
Platonic Solids A
Fig. 16.21 B
The regular, convex polyhedra are known as the platonic solids (Fig. 16.22). There
are only five platonic solids. In a platonic solid,
(i) at least three faces meet at a vertex to form a solid angle.
(ii) the sum of all plane angles forming the solid angle at a vertex is less than 360º.

The five platonic solids are:
(a) Tetrahedron: It is also called triangular pyramid. Its faces are congruent equilateral triangles.
(b) Hexahedron: It is also called cube. Its faces are 6 squares.

248