Page 254 - Start Up Mathematics_8 (Non CCE)

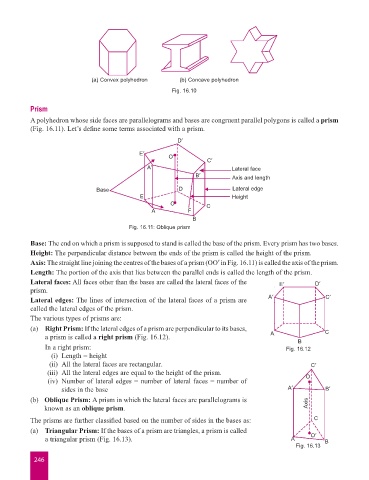
(a) Convex polyhedron (b) Concave polyhedron
Fig. 16.10

Prism
A polyhedron whose side faces are parallelograms and bases are congruent parallel polygons is called a prism
(Fig. 16.11). Let’s define some terms associated with a prism.
D¢

E¢
O¢
C¢
A¢ Lateral face
B¢ Axis and length

Base D Lateral edge
E Height
O C
A F
B
Fig. 16.11: Oblique prism

Base: The end on which a prism is supposed to stand is called the base of the prism. Every prism has two bases.
Height: The perpendicular distance between the ends of the prism is called the height of the prism.
Axis: The straight line joining the centres of the bases of a prism (OO¢ in Fig. 16.11) is called the axis of the prism.
Length: The portion of the axis that lies between the parallel ends is called the length of the prism.
Lateral faces: All faces other than the bases are called the lateral faces of the E¢ D¢
prism.
Lateral edges: The lines of intersection of the lateral faces of a prism are A¢ C¢
called the lateral edges of the prism. B¢
The various types of prisms are: E D

(a) Right Prism: If the lateral edges of a prism are perpendicular to its bases, C
a prism is called a right prism (Fig. 16.12). A B
In a right prism: Fig. 16.12
(i) Length = height
(ii) All the lateral faces are rectangular. C¢
(iii) All the lateral edges are equal to the height of the prism. O
(iv) Number of lateral edges = number of lateral faces = number of
sides in the base A¢ B¢
(b) Oblique Prism: A prism in which the lateral faces are parallelograms is Axis
known as an oblique prism.
The prisms are further classified based on the number of sides in the bases as: C
(a) Triangular Prism: If the bases of a prism are triangles, a prism is called
a triangular prism (Fig. 16.13). A O¢ B
Fig. 16.13
246