Page 122 - Start Up Mathematics_8 (Non CCE)

2
2
3
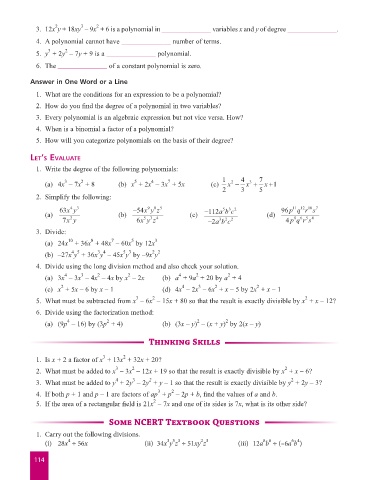
3. 12x y + 18xy – 9x + 6 is a polynomial in ______________ variables x and y of degree ______________.
4. A polynomial cannot have ______________ number of terms.
3
2
5. y + 2y – 7y + 9 is a ______________ polynomial.
6. The ______________ of a constant polynomial is zero.

Answer in One Word or a Line

1. What are the conditions for an expression to be a polynomial?
2. How do you find the degree of a polynomial in two variables?
3. Every polynomial is an algebraic expression but not vice versa. How?

4. When is a binomial a factor of a polynomial?
5. How will you categorize polynomials on the basis of their degree?

let’s evaluate
1. Write the degree of the following polynomials:

4
7
5
3
2
2
3
(a) 4x – 7x + 8 (b) x + 2x – 3x + 5x (c) 1 x - 4 x + 7 x + 1
2 3 5
2. Simplify the following:
11 12 10 7
4
63xy 3 -54xy z -112ab c 96pq rs
9
8 5
53 2
(a) (b) (c) (d)
5
3 4
86 54
2
7xy 6xy z -2ab c 4pq rs
32 2
3. Divide:
8
10
5
7
(a) 24x + 36x + 48x – 60x by 12x 3
2 2
3 4
5 3
4 5
(b) –27x y + 36x y – 45x y by –9x y
4. Divide using the long division method and also check your solution.
2
2
4
2
2
4
3
(a) 3x – 3x – 4x – 4x by x – 2x (b) a + 9a + 20 by a + 4
2
2
3
4
2
(c) x + 5x – 6 by x – 1 (d) 4x – 2x – 6x + x – 5 by 2x + x – 1
2
2
3
5. What must be subtracted from x – 6x – 15x + 80 so that the result is exactly divisible by x + x – 12?
6. Divide using the factorization method:
2
4
2
2
(a) (9p – 16) by (3p + 4) (b) (3x – y) – (x + y) by 2(x – y)
Thinking Skills
2
3
1. Is x + 2 a factor of x + 13x + 32x + 20?
3
2
2
2. What must be added to x – 3x – 12x + 19 so that the result is exactly divisible by x + x – 6?
2
2
3
4
3. What must be added to y + 2y – 2y + y – 1 so that the result is exactly divisible by y + 2y – 3?
2
3
4. If both p + 1 and p – 1 are factors of ap + p – 2p + b, find the values of a and b.
2
5. If the area of a rectangular field is 21x – 7x and one of its sides is 7x, what is its other side?
Some NCERT Textbook Questions
1. Carry out the following divisions.
6 4
2 3
4
3 3 3
8 8
(i) 28x ÷ 56x (ii) 34x y z ÷ 51xy z (iii) 12a b ÷ (–6a b )
114