Page 119 - Start Up Mathematics_8 (Non CCE)

2
3
3
4
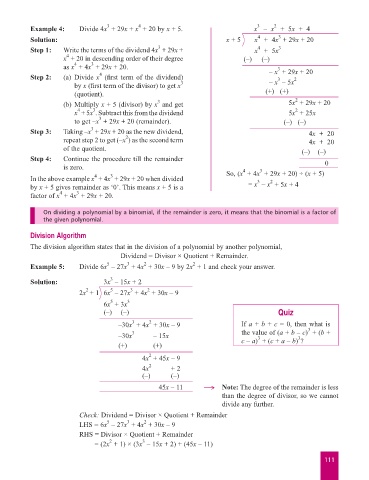
Example 4: Divide 4x + 29x + x + 20 by x + 5. x – x + 5x + 4
3
4
Solution: x + 5 x + 4x + 29x + 20
3
4
Step 1: Write the terms of the dividend 4x + 29x + x + 5x 3
4
x + 20 in descending order of their degree (–) (–)
4
3
as x + 4x + 29x + 20. – x + 29x + 20
3
4
Step 2: (a) Divide x (first term of the dividend) 3 2
3
by x (first term of the divisor) to get x – x – 5x
(quotient). (+) (+)
2
3
(b) Multiply x + 5 (divisor) by x and get 5x + 29x + 20
3
2
4
x + 5x . Subtract this from the dividend 5x + 25x
3
to get –x + 29x + 20 (remainder). (–) (–)
3
Step 3: Taking –x + 29x + 20 as the new dividend, 4x + 20
2
repeat step 2 to get (–x ) as the second term 4x + 20
of the quotient. (–) (–)
Step 4: Continue the procedure till the remainder
is zero. 4 3 0
3
4
In the above example x + 4x + 29x + 20 when divided So, (x + 4x + 29x + 20) ÷ (x + 5)
3
2
by x + 5 gives remainder as ‘0’. This means x + 5 is a = x – x + 5x + 4
3
4
factor of x + 4x + 29x + 20.
On dividing a polynomial by a binomial, if the remainder is zero, it means that the binomial is a factor of
the given polynomial.
Division Algorithm
The division algorithm states that in the division of a polynomial by another polynomial,
Dividend = Divisor × Quotient + Remainder.
5
3
2
2
Example 5: Divide 6x – 27x + 4x + 30x – 9 by 2x + 1 and check your answer.
3
Solution: 3x – 15x + 2
5
2
2
3
2x + 1 6x – 27x + 4x + 30x – 9
5
6x + 3x 3
(–) (–) Quiz
3
2
–30x + 4x + 30x – 9 If a + b + c = 0, then what is
3
3
–30x – 15x the value of (a + b – c) + (b +
3
3
(+) (+) c – a) + (c + a – b) ?
2
4x + 45x – 9
2
4x + 2
(–) (–)
45x – 11 Æ Note: The degree of the remainder is less
than the degree of divisor, so we cannot
divide any further.
Check: Dividend = Divisor × Quotient + Remainder
3
5
2
LHS = 6x – 27x + 4x + 30x – 9
RHS = Divisor × Quotient + Remainder
2
3
= (2x + 1) × (3x – 15x + 2) + (45x – 11)
111