Page 120 - Start Up Mathematics_8 (Non CCE)

2
3
3
= 2x × (3x – 15x + 2) + 1 × (3x – 15x + 2) + (45x – 11)
5
3
2
3
= 6x – 30x + 4x + 3x – 15x + 2 + 45x – 11
3
5
2
= 6x – 27x + 4x + 30x – 9
= LHS
5
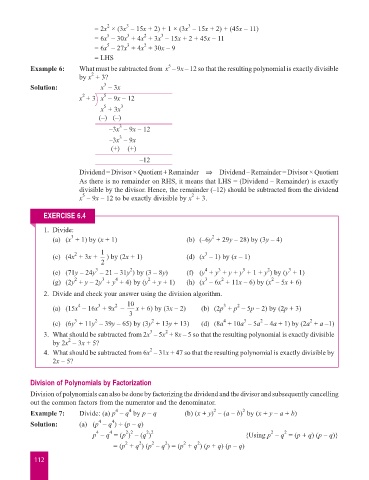
Example 6: What must be subtracted from x – 9x – 12 so that the resulting polynomial is exactly divisible
2
by x + 3?
3
Solution: x – 3x
2
5
x + 3 x – 9x – 12
5
x + 3x 3
(–) (–)
3
–3x – 9x – 12
3
–3x – 9x
(+) (+)
–12
Dividend = Divisor × Quotient + Remainder fi Dividend – Remainder = Divisor × Quotient
As there is no remainder on RHS, it means that LHS = (Dividend – Remainder) is exactly
divisible by the divisor. Hence, the remainder (–12) should be subtracted from the dividend
2
5
x – 9x – 12 to be exactly divisible by x + 3.
EXERCISE 6.4

1. Divide:
3
2
(a) (x + 1) by (x + 1) (b) (–6y + 29y – 28) by (3y – 4)
3
2
(c) (4x + 3x + 1 ) by (2x + 1) (d) (x – 1) by (x – 1)
2
2
2
3
4
3
5
3
(e) (71y – 24y – 21 – 31y ) by (3 – 8y) (f) (y + y + y + y + 1 + y ) by (y + 1)
4
2
3
2
3
2
2
(g) (2y + y – 2y + y + 4) by (y + y + 1) (h) (x – 6x + 11x – 6) by (x – 5x + 6)
2. Divide and check your answer using the division algorithm.
3
4
2
3
2
(a) (15x – 16x + 9x – 10 x + 6) by (3x – 2) (b) (2p + p – 5p – 2) by (2p + 3)
3
3
2
2
4
2
2
3
(c) (6y + 11y – 39y – 65) by (3y + 13y + 13) (d) (8a + 10a – 5a – 4a + 1) by (2a + a –1)
3
2
3. What should be subtracted from 2x – 5x + 8x – 5 so that the resulting polynomial is exactly divisible
2
by 2x – 3x + 5?
2
4. What should be subtracted from 6x – 31x + 47 so that the resulting polynomial is exactly divisible by
2x – 5?
Division of Polynomials by Factorization
Division of polynomials can also be done by factorizing the dividend and the divisor and subsequently cancelling
out the common factors from the numerator and the denominator.
2
4
2
4
Example 7: Divide: (a) p – q by p – q (b) (x + y) – (a – b) by (x + y – a + b)
4
4
Solution: (a) (p – q ) ÷ (p – q)
2
2
2 2
2 2
4
4
p – q = (p ) – (q ) {Using p – q = (p + q) (p – q)}
2
2
2
2
2
2
= (p + q ) (p – q ) = (p + q ) (p + q) (p – q)
112