Page 24 - ICSE Math 8

1
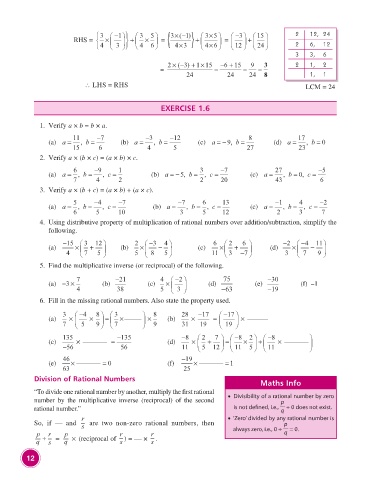
 3  − 1  3 5  { 3×−()  35×   3  15  2 12, 24
− 
RHS =  ×   +  ×  = }   =   +  
+
×
 4  3   4 6  43×  46   12   24  2 6, 12
3 3, 6
615
2 ×−( 3 +×) 115 −+ 9 3 2 1, 2
= = = =
24 24 24 8 1, 1
∴ LHS = RHS LCM = 24

EXERCISE 1.6

1. Verify a × b = b × a.
11 −7 −3 −12 8 17
(a) a = , b = (b) a = , b = (c) a =−9, b = (d) a = , b = 0
15 6 4 5 27 23
2. Verify a × (b × c) = (a × b) × c.

6 −9 1 3 −7 27 −5
(a) a = , b = , c = (b) a =−5, b = , c = (c) a = , b = 0, c =
7 4 2 2 20 43 6
3. Verify a × (b + c) = (a × b) + (a × c).

5 −4 −7 −7 6 13 −1 4 −2
(a) a = , b = , c = (b) a = , b = , c = (c) a = , b = , c =
6 5 10 3 5 12 2 3 7
4. Using distributive property of multiplication of rational numbers over addition/subtraction, simplify the
following.

−15  3 12  2  − 3 4  6  2 6  −2  −4 11 
(a) ×  +  (b) ×  −  (c) ×  +  (d) ×  − 
4  7 5  5  8 5  11  3 − 7  3  7 9 

5. Find the multiplicative inverse (or reciprocal) of the following.

7 −21 4  − 2  75 −30
(a) −×3 (b) (c) ×   (d) (e) (f) –1
4 38 5  3  − 63 −19
6. Fill in the missing rational numbers. Also state the property used.

3  − 4 8   3 _____  8 28 − 17  − 17  ______
(a) ×  ×   ×  × (b) × =   ×
=
7  5 9   7  9 31 19  19 
135 _______ − 135 −8  2 7   −8 2   −8 _______ 
=
(c) × = (d) ×  +   ×  +  × 
− 56 56 11  5 12   11 5   11 
46 _______ −19 _______
(e) × = 0 (f) × =1
63 25
Division of Rational Numbers
Maths Info
“To divide one rational number by another, multiply the first rational
number by the multiplicative inverse (reciprocal) of the second • Divisibility of a rational number by zero
p
rational number.” is not defined, i.e., ÷ 0 does not exist.
q
p r • ‘Zero’ divided by any rational number is
So, if q and s are two non-zero rational numbers, then p
q
p r p r p r always zero, i.e., 0 ÷ = 0.
s
q ÷ s = q × (reciprocal of ) = q × .
s
12