Page 29 - ICSE Math 8

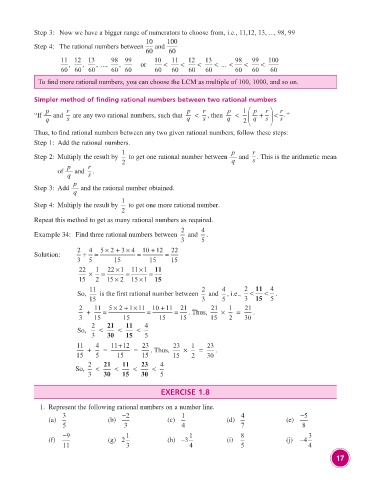
Step 3: Now we have a bigger range of numerators to choose from, i.e., 11,12, 13, ..., 98, 99
10 100
Step 4: The rational numbers between and
60 60
11 12 13 98 99 10 11 12 13 98 99 100
, , , ..., , or < < < < ... < < <
60 60 60 60 60 60 60 60 60 60 60 60
To find more rational numbers, you can choose the LCM as multiple of 100, 1000, and so on.

Simpler method of finding rational numbers between two rational numbers
p r p r p 1  p r  r
“If and are any two rational numbers, such that < , then <  +  < . ”
q s q s q 2  q s  s
Thus, to find rational numbers between any two given rational numbers, follow these steps:
Step 1: Add the rational numbers.
1 p r
Step 2: Multiply the result by to get one rational number between q and . This is the arithmetic mean
s
p r 2
of and .
q s
p
Step 3: Add and the rational number obtained.
q
1
Step 4: Multiply the result by to get one more rational number.
2
Repeat this method to get as many rational numbers as required.
2 4
Example 34: Find three rational numbers between and .
3 5

2 4 52× + 34× 10 12+ 22
Solution: + = = =
3 5 15 15 15
22 1 22 1× 11 1× 11
× = = =
15 2 15 2× 15 1× 15
11 2 4 2 11 4
So, is the first rational number between and , i.e., < < .
15 3 5 3 15 5
2 11 52 111 10 11× + × + 21 21 1 21
+ = = = . Thus, × = .
3 15 15 15 15 15 2 30
2 21 11 4
So, < < <
3 30 15 5
11 4 11 12+ 23 23 1 23
+ = = . Thus, × = .
15 5 15 15 15 2 30
2 21 11 23 4
So, < < < <
3 30 15 30 5

EXERCISE 1.8

1. Represent the following rational numbers on a number line.
3 −2 1 4 −5
(a) (b) (c) (d) (e)
5 3 4 7 8
−9 1 1 8 3
(f) (g) 2 (h) −3 (i) (j) −4
11 3 4 5 4

17