Page 28 - ICSE Math 8

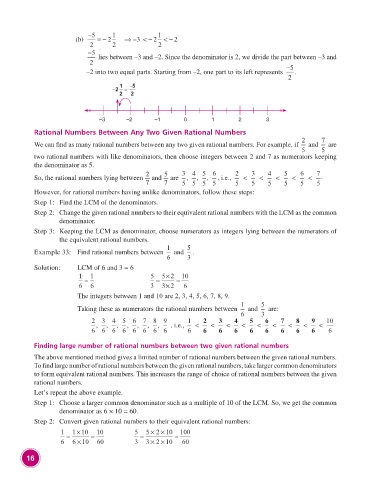
−5 1 1
(b) =− 2 ⇒ –3 <− 2 <− 2
2 2 2
−5 lies between –3 and –2. Since the denominator is 2, we divide the part between –3 and
2
–2 into two equal parts. Starting from –2, one part to its left represents −5 .
2
1 −5
−2 =
2 2

–3 –2 –1 0 1 2 3

Rational Numbers Between Any Two Given Rational Numbers
2 7
We can find as many rational numbers between any two given rational numbers. For example, if and are
5 5
two rational numbers with like denominators, then choose integers between 2 and 7 as numerators keeping
the denominator as 5.
3 4 5 6
5
2
So, the rational numbers lying between and are , , , , i.e., 2 < 3 < 4 < 5 < 6 < 7 .
7 7 5 5 5 5 5 5 5 5 5 5
However, for rational numbers having unlike denominators, follow these steps:
Step 1: Find the LCM of the denominators.
Step 2: Change the given rational numbers to their equivalent rational numbers with the LCM as the common
denominator.

Step 3: Keeping the LCM as denominator, choose numerators as integers lying between the numerators of
the equivalent rational numbers.
1 5
Example 33: Find rational numbers between and .
6 3
Solution: LCM of 6 and 3 = 6
1 1 5 52× 10
= = =
6 6 3 32× 6
The integers between 1 and 10 are 2, 3, 4, 5, 6, 7, 8, 9.
1 5
Taking these as numerators the rational numbers between and are:
6 3
2 3 4 5 6 7 8 9 , i.e., 1 < 2 < 3 < 4 < 5 < 6 < 7 < 8 < 9 < 10
,
,
,
,
,
,
,
6 6 6 6 6 6 6 6 6 6 6 6 6 6 6 6 6 6
Finding large number of rational numbers between two given rational numbers
The above mentioned method gives a limited number of rational numbers between the given rational numbers.
To find large number of rational numbers between the given rational numbers, take larger common denominators
to form equivalent rational numbers. This increases the range of choice of rational numbers between the given
rational numbers.
Let’s repeat the above example.
Step 1: Choose a larger common denominator such as a multiple of 10 of the LCM. So, we get the common
denominator as 6 × 10 = 60.
Step 2: Convert given rational numbers to their equivalent rational numbers:
1 110× 10 5 52 10×× 100
= = = =
6 610× 60 3 32 10×× 60

16