Page 191 - ICSE Math 8

17 Construction of Quadrilaterals

Key Concepts

• Construction of Quadrilaterals: Required Conditions • Construction of a Quadrilateral Given 3 Sides and
• Construction of a Quadrilateral Given 4 Sides and a 2 Included Angles
Diagonal • Construction of a Quadrilateral Given 3 angles and
• Construction of a Quadrilateral Given 3 Sides and 2 Sides
2 Diagonals • Construction of Some Special Quadrilaterals
• Construction of a Quadrilateral Given 4 Sides and an
Angle

We have already learnt about construction of triangles in the previous class. A triangle consists of three sides
and three angles. To construct a triangle, we require three measures of sides or angles (with at least one
given side). A quadrilateral is a polygon with 4 sides, 4 enclosed angles and 2 diagonals. So, in all there are
10 building blocks of a quadrilateral. Can you tell how many building blocks are required to draw a convex
quadrilateral?

Construction of Quadrilaterals: Required Conditions

To construct a convex quadrilateral, at least five building blocks are required.
In this chapter, we will learn to construct a convex quadrilateral with the following conditions:
(a) When the measures of 4 sides and 1 diagonal are given.
(b) When the measures of 3 sides and 2 diagonals are given.
(c) When the measures of 4 sides and 1 enclosed angle are given.
(d) When the measures of 3 sides and 2 enclosed angles are given.
(e) When the measures of 2 sides and 3 enclosed angles are given.

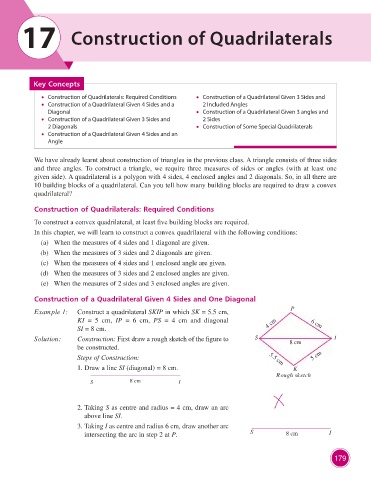
Construction of a Quadrilateral Given 4 Sides and One Diagonal
Example 1: Construct a quadrilateral SKIP in which SK = 5.5 cm, P
KI = 5 cm, IP = 6 cm, PS = 4 cm and diagonal 4 cm 6 cm
SI = 8 cm.
Solution: Construction: First draw a rough sketch of the figure to S 8 cm I
be constructed.
Steps of Construction: 5.5 cm 5 cm
1. Draw a line SI (diagonal) = 8 cm. K
Rough sketch
S 8 cm I

P
2. Taking S as centre and radius = 4 cm, draw an arc
above line SI.
3. Taking I as centre and radius 6 cm, draw another arc
intersecting the arc in step 2 at P. S 8 cm I

179