Page 148 - ICSE Math 8

13 Algebraic Identities

Key Concepts

2
2
• Standard Identities: (a + b) = a + 2ab + b 2 • Special Products
2
2
(a – b) = a – 2ab + b 2
2
2
a – b = (a – b)(a + b)
An algebraic identity is a statement of equality between two algebraic expressions that is satisfied for all the
2
values of the variables. For example, (x – 3)(x + 2) ∫ x – x – 6 is satisfied for all values of x. The sign ‘∫’ is
used to distinguish an identity from an equation.
Standard Identities

2
2
Identity 1: (a + b) = a + 2ab + b 2
In other words,
2
2
(Sum of the two terms) = (First term) + 2 ¥ (First term) ¥ (Second term) + (Second term) 2
2
Proof: (a + b) = (a + b)(a + b)
= a(a + b) + b(a + b) (Distributive property of multiplication over addition)
2
= a + ab + ab + b 2
2
2
= a + 2ab + b (Commutative property ab = ba)
2
2
\ (a + b) = a + 2ab + b 2
Verification
2
2
2
Let us verify the identity (a + b) = a + 2ab + b by performing the following activity.
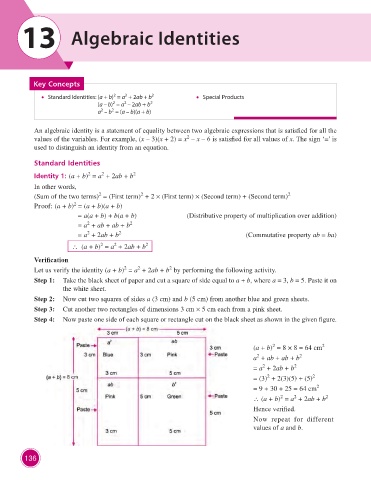
Step 1: Take the black sheet of paper and cut a square of side equal to a + b, where a = 3, b = 5. Paste it on
the white sheet.
Step 2: Now cut two squares of sides a (3 cm) and b (5 cm) from another blue and green sheets.
Step 3: Cut another two rectangles of dimensions 3 cm ¥ 5 cm each from a pink sheet.
Step 4: Now paste one side of each square or rectangle cut on the black sheet as shown in the given figure.

2
(a + b) = 8 × 8 = 64 cm 2
2
a + ab + ab + b 2
2
= a + 2ab + b 2
2
= (3) + 2(3)(5) + (5) 2
= 9 + 30 + 25 = 64 cm 2
2
2
\ (a + b) = a + 2ab + b 2
Hence verified.
Now repeat for different
values of a and b.

136