Page 144 - ICSE Math 8

Step 5: Repeat steps 3 and 4 till the remainder is zero or a polynomial of degree lower than that of the divisor.
We can verify the result of division by using the formula:
Dividend = Divisor × Quotient + Remainder
3
2
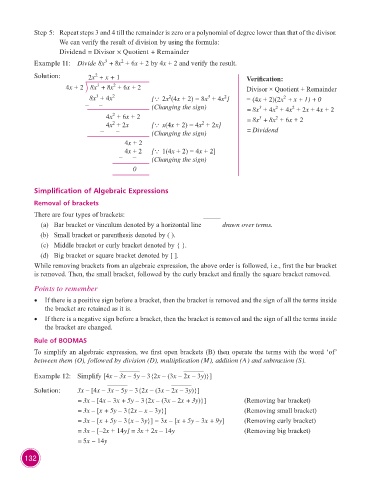
Example 11: Divide 8x + 8x + 6x + 2 by 4x + 2 and verify the result.
Solution: 2x + x + 1 Verification:
2
3
2
4x + 2 8x + 8x + 6x + 2 Divisor × Quotient + Remainder
3
3
2
8x + 4x 2 [ 2x (4x + 2) = 8x + 4x ] = (4x + 2)(2x + x + 1) + 0
2
2
– – (Changing the sign) = 8x + 4x + 4x + 2x + 4x + 2
3
2
2
2
4x + 6x + 2 = 8x + 8x + 6x + 2
2
3
4x + 2x [ x(4x + 2) = 4x + 2x]
2
2
– – (Changing the sign) = Dividend
4x + 2
4x + 2 [ 1(4x + 2) = 4x + 2]
– – (Changing the sign)
0
Simplification of Algebraic Expressions
Removal of brackets
There are four types of brackets: _____
(a) Bar bracket or vinculum denoted by a horizontal line drawn over terms.
(b) Small bracket or parenthesis denoted by ( ).
(c) Middle bracket or curly bracket denoted by { }.

(d) Big bracket or square bracket denoted by [ ].
While removing brackets from an algebraic expression, the above order is followed, i.e., first the bar bracket
is removed. Then, the small bracket, followed by the curly bracket and finally the square bracket removed.

Points to remember

• If there is a positive sign before a bracket, then the bracket is removed and the sign of all the terms inside
the bracket are retained as it is.
• If there is a negative sign before a bracket, then the bracket is removed and the sign of all the terms inside
the bracket are changed.
Rule of BODMAS

To simplify an algebraic expression, we first open brackets (B) then operate the terms with the word ‘of’
between them (O), followed by division (D), multiplication (M), addition (A) and subtraction (S).

Example 12: Simplify [4x – 3x – 5y – 3{2x – (3x – 2x – 3y)}]

Solution: 3x – [4x – 3x – 5y – 3{2x – (3x – 2x – 3y)}]
= 3x – [4x – 3x + 5y – 3{2x – (3x – 2x + 3y)}] (Removing bar bracket)
= 3x – [x + 5y – 3{2x – x – 3y}] (Removing small bracket)
= 3x – [x + 5y – 3{x – 3y}] = 3x – [x + 5y – 3x + 9y] (Removing curly bracket)
= 3x – [–2x + 14y] = 3x + 2x – 14y (Removing big bracket)
= 5x – 14y

132