Page 79 - Start Up Mathematics_8 (Non CCE)

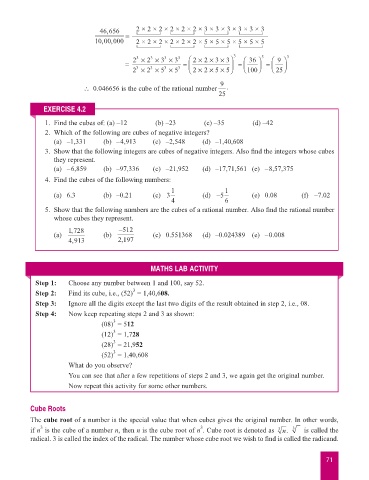
46 656, 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3 × 3 × 3 × 3 × 3

=
10 00 000, , 2 × 2 × 2 × 2 × 2 × 2 × 5 × 5 × 5 × 5 × 5 × 5

3
3
3
3
2 ¥ 2 ¥ 3 ¥ 3 3 Ê 2 ¥ 2 ¥ ¥ 3ˆ 3 Ê 36 ˆ 3 Ê 9 9 ˆ 3
= = = Á ˜ = Á ˜
2 ¥ 2 ¥ 5 ¥ 5 3 Á Ë 2 ¥ 2 ¥ 5 ¥ 5¯ ˜ Ë 100¯ Ë 25¯
3
3
3
9
\ 0.046656 is the cube of the rational number .
25
EXERCISE 4.2
1. Find the cubes of: (a) –12 (b) –23 (c) –35 (d) –42
2. Which of the following are cubes of negative integers?
(a) –1,331 (b) –4,913 (c) –2,548 (d) –1,40,608
3. Show that the following integers are cubes of negative integers. Also find the integers whose cubes
they represent.
(a) –6,859 (b) –97,336 (c) –21,952 (d) –17,71,561 (e) –8,57,375
4. Find the cubes of the following numbers:
1 1
(a) 6.3 (b) –0.21 (c) 3 (d) -5 (e) 0.08 (f) –7.02
4 6
5. Show that the following numbers are the cubes of a rational number. Also find the rational number
whose cubes they represent.

1 728, -512
(a) (b) (c) 0.551368 (d) –0.024389 (e) –0.008
4 913, 2 197,

MATHS LAB ACTIVITY

Step 1: Choose any number between 1 and 100, say 52.
3
Step 2: Find its cube, i.e., (52) = 1,40,608.
Step 3: Ignore all the digits except the last two digits of the result obtained in step 2, i.e., 08.
Step 4: Now keep repeating steps 2 and 3 as shown:
3
(08) = 512
3
(12) = 1,728
3
(28) = 21,952
3
(52) = 1,40,608
What do you observe?
You can see that after a few repetitions of steps 2 and 3, we again get the original number.
Now repeat this activity for some other numbers.

Cube Roots
The cube root of a number is the special value that when cubes gives the original number. In other words,
3
3
3
if n is the cube of a number n, then n is the cube root of n . Cube root is denoted as n. 3 is called the
radical. 3 is called the index of the radical. The number whose cube root we wish to find is called the radicand.
71