Page 76 - Start Up Mathematics_8 (Non CCE)

3
3
3
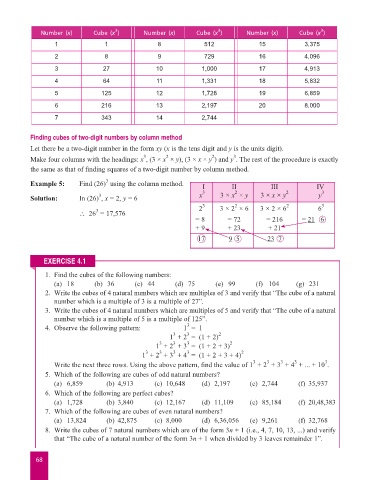
Number (x) Cube (x ) Number (x) Cube (x ) Number (x) Cube (x )
1 1 8 512 15 3,375
2 8 9 729 16 4,096
3 27 10 1,000 17 4,913

4 64 11 1,331 18 5,832

5 125 12 1,728 19 6,859
6 216 13 2,197 20 8,000
7 343 14 2,744

Finding cubes of two-digit numbers by column method

Let there be a two-digit number in the form xy (x is the tens digit and y is the units digit).
2
3
3
2
Make four columns with the headings: x , (3 × x × y), (3 × x × y ) and y . The rest of the procedure is exactly
the same as that of finding squares of a two-digit number by column method.
3
Example 5: Find (26) using the column method. I II III IV

2
2
3

3
Solution: In (26) , x = 2, y = 6 x 3 × x × y 3 × x × y y 3
3
3
2
2
3
\ 26 = 17,576 2 3 × 2 × 6 3 × 2 × 6 6
= 8 = 72 = 216 = 21 6
+ 9 + 23 + 21
17 9 5 23 7
EXERCISE 4.1
1. Find the cubes of the following numbers:
(a) 18 (b) 36 (c) 44 (d) 75 (e) 99 (f) 104 (g) 231
2. Write the cubes of 4 natural numbers which are multiples of 3 and verify that “The cube of a natural
number which is a multiple of 3 is a multiple of 27”.
3. Write the cubes of 4 natural numbers which are multiples of 5 and verify that “The cube of a natural
number which is a multiple of 5 is a multiple of 125”.
3
4. Observe the following pattern: 1 = 1
3
3
1 + 2 = (1 + 2) 2
3
3
3
1 + 2 + 3 = (1 + 2 + 3) 2
3
3
3
3
1 + 2 + 3 + 4 = (1 + 2 + 3 + 4) 2
3
3
3
3
3
Write the next three rows. Using the above pattern, find the value of 1 + 2 + 3 + 4 + ... + 10 .
5. Which of the following are cubes of odd natural numbers?
(a) 6,859 (b) 4,913 (c) 10,648 (d) 2,197 (e) 2,744 (f) 35,937
6. Which of the following are perfect cubes?
(a) 1,728 (b) 3,840 (c) 12,167 (d) 11,109 (e) 85,184 (f) 20,48,383
7. Which of the following are cubes of even natural numbers?
(a) 13,824 (b) 42,875 (c) 8,000 (d) 6,36,056 (e) 9,261 (f) 32,768
8. Write the cubes of 7 natural numbers which are of the form 3n + 1 (i.e., 4, 7, 10, 13, ...) and verify
that “The cube of a natural number of the form 3n + 1 when divided by 3 leaves remainder 1”.
68