Page 75 - Start Up Mathematics_8 (Non CCE)

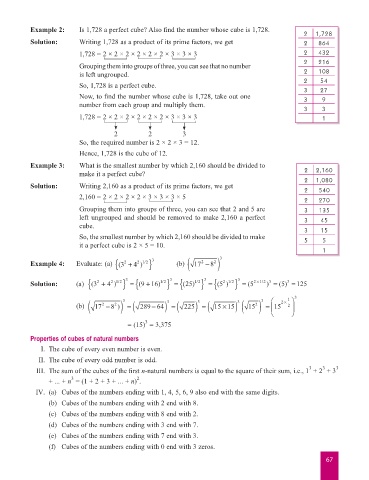
Example 2: Is 1,728 a perfect cube? Also find the number whose cube is 1,728.
2 1,728
Solution: Writing 1,728 as a product of its prime factors, we get 2 864
1,728 = 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3 × 3 2 432
2 216
Grouping them into groups of three, you can see that no number
is left ungrouped. 2 108
So, 1,728 is a perfect cube. 2 54
3
27
Now, to find the number whose cube is 1,728, take out one 3 9
number from each group and multiply them.
3 3
1,728 = 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3 × 3 1

2 2 3
So, the required number is 2 × 2 × 3 = 12.

Hence, 1,728 is the cube of 12.
Example 3: What is the smallest number by which 2,160 should be divided to
make it a perfect cube? 2 2,160
2 1,080
Solution: Writing 2,160 as a product of its prime factors, we get 2 540
2,160 = 2 × 2 × 2 × 2 × 3 × 3 × 3 × 5 2 270

Grouping them into groups of three, you can see that 2 and 5 are 3 135
left ungrouped and should be removed to make 2,160 a perfect 3 45
cube. 3 15

So, the smallest number by which 2,160 should be divided to make 5 5
it a perfect cube is 2 × 5 = 10.
1
{
Example 4: Evaluate: (a) (3 + 4 2 ) } 3 (b) ( 17 - 8 2 ) 3
2
2
/ 12
{
3
3
3
3
/ 12
Solution: (a) (3 + 4 2 ) } = { (916+ ) } = { (25 } = { ()5 } = (5 21¥ /23 3 =125
21
/2
/ 12
/ 12
2
)
) == ()5
( 3 ) ( ) ( ) ( ) 3 Ê 2 ¥ 1 ˆ 3
3
3
3
(b) 17 - 8 ) ) ( 289 64- = 225 = 15 15¥ 15 2 = Á 15 2 ˜
2
2
=
Ë ¯
3
= (15) = 3,375
Properties of cubes of natural numbers
I. The cube of every even number is even.
II. The cube of every odd number is odd.
3
3
3
III. The sum of the cubes of the first n-natural numbers is equal to the square of their sum, i.e., 1 + 2 + 3
3
2
+ ... + n = (1 + 2 + 3 + ... + n) .
IV. (a) Cubes of the numbers ending with 1, 4, 5, 6, 9 also end with the same digits.
(b) Cubes of the numbers ending with 2 end with 8.
(c) Cubes of the numbers ending with 8 end with 2.
(d) Cubes of the numbers ending with 3 end with 7.
(e) Cubes of the numbers ending with 7 end with 3.
(f) Cubes of the numbers ending with 0 end with 3 zeros.
67