Page 81 - Start Up Mathematics_8 (Non CCE)

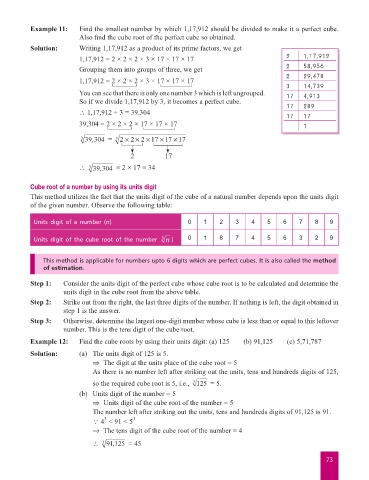
Example 11: Find the smallest number by which 1,17,912 should be divided to make it a perfect cube.
Also find the cube root of the perfect cube so obtained.
Solution: Writing 1,17,912 as a product of its prime factors, we get
1,17,912 = 2 × 2 × 2 × 3 × 17 × 17 × 17 2 1,17,912
Grouping them into groups of three, we get 2 58,956
2 29,478
1,17,912 = 2 × 2 × 2 × 3 × 17 × 17 × 17
3 14,739
You can see that there is only one number 3 which is left ungrouped. 17 4,913
So if we divide 1,17,912 by 3, it becomes a perfect cube. 17 289
\ 1,17,912 ÷ 3 = 39,304
17 17
39,304 = 2 × 2 × 2 × 17 × 17 × 17 1

39 304, = 2 ¥¥ ¥ ¥
2 2171717¥
3
3
2 17
\ 39 304, = 2 × 17 = 34
3

Cube root of a number by using its units digit
This method utilizes the fact that the units digit of the cube of a natural number depends upon the units digit
of the given number. Observe the following table:

Units digit of a number (n) 0 1 2 3 4 5 6 7 8 9

3
Units digit of the cube root of the number n ) 0 1 8 7 4 5 6 3 2 9

This method is applicable for numbers upto 6 digits which are perfect cubes. It is also called the method
of estimation.

Step 1: Consider the units digit of the perfect cube whose cube root is to be calculated and determine the
units digit in the cube root from the above table.
Step 2: Strike out from the right, the last three digits of the number. If nothing is left, the digit obtained in
step 1 is the answer.
Step 3: Otherwise, determine the largest one-digit number whose cube is less than or equal to this leftover
number. This is the tens digit of the cube root.
Example 12: Find the cube roots by using their units digit: (a) 125 (b) 91,125 (c) 5,71,787
Solution: (a) The units digit of 125 is 5.
fi The digit at the units place of the cube root = 5
As there is no number left after striking out the units, tens and hundreds digits of 125,

3
so the required cube root is 5, i.e., 125 = 5.
(b) Units digit of the number = 5
fi Units digit of the cube root of the number = 5
The number left after striking out the units, tens and hundreds digits of 91,125 is 91.
3
 4 < 91 < 5 3
fi The tens digit of the cube root of the number = 4

\ 91125, = 45
3
73